XYLENE POWER LTD.

ELECTROMAGNETIC SPHEROMAK

By Charles Rhodes, P.Eng., Ph.D.

TOROIDAL MAGNETIC FIELD ENERGY:
The toroidal magnetic field energy is:
Integral from R = Rc to R = Rs of:
Uto (Ro / R)^2 2 Pi R dR (2 Zs)
= Integral from R = Rc to R = Rs of:
Uto (Ro / R)^2 2 Pi R dR (2 [Ho^2 (R / Ro) - (Ro - R)^2]^0.5)
= Integral from R = Rc to R = Rs of:
Uto (Ro^2 / R) 4 Pi dR ([Ho^2 (R / Ro) - (Ro - R)^2]^0.5)
which may need numerical integration.

Note that 113 is prime and 355 = 5(71). Ideally for highly stable spheromaks both Np and Nt should be prime. However, if 113 is disturbed to either 112 = (7 X 2 X 2 X 2 X 2) or to 114 = (107 X 2) it still shares no common factors with 355, which potentially provides the required spheromak stability.

This expression is used on the web page titled: PLANCK CONSTANT to determine the Planck Constant and the Fine Structure Constant.

*****************************************************

***************************************************

Define:
F = C / Lh

Hence:
Upo = [Muo / 2] [F Qs Np / 2 Ro]^2
 

SPHEROMAK END CONDITIONS:
The spheromak ends are mirror images of each other. Let Rf be the radius of the spheromak end funnel face at the spheromak's longest point. Then the following boundary conditions apply outside the spheromak end face:
For R < Rc the spheromak has no physical end and the magnetic field is entirely poloidal;

For Rc < R < Rs and |Z| < |Zs| the internal magnetic field Bti is toroidal;
For Rc < R < Rs and |Z| < |Zs| the magnetic field Bti is proportional to (1 / R).

Outside the spheromak wall the magnetic field is purely poloidal;
For Rc < R < Rs and Z^2 < Zs^2 the electic field component parallel to the main axis of symmetry is zero;
For (A^2 R^2 + B^2 Z^2) >> Zf^2 the electric field is spherically radial;
For (A^2 R^2 + B^2 Z^2) >> Zf^2 spherical electric field is proportional to (A^2 R^2 + B^2 Z^2)^-1;
 

When these constraints are properly applied the quantitative agreement between the engineering model and published spheromak photographs is remarkable.
 

************************************************************

ELECTROMAGNETIC SPHEROMAK STRUCTURE:
1) A spheromak wall is composed of a closed spiral of charge hose or plasma hose of overall length Lh;

2) Spheromak net charge Qs is uniformly distributed over charge hose or plasma hose length Lh resulting in a net charge per unit length:
[Qs / Lh];

3) The spheromak net charge circulates at the speed of light C (constant velocity) along the charge hose path, which gives the spheromak a natural frequency:
Fh = C / Lh

4) The flow of net charge Ih has two orthogonal charge circulation velocity components, a component which contributes to the external poloidal magnetic field and a component which contributes to the internal toroidal magnetic field. The orthogonal current flows can each be either positive or negative, so a spheromak has 4 possible quantum states relate to an observer. Hence each spheromak has two orthogonal magnetic vectors (poloidal and toroidal) each of which has two possible orientations. There is poloidal up and poloidal down magnetic vector. For each of the two possible poloidal magnetic vector directions there is toroidal clockwise (CW) and toroidal counter clockwise (CCW) magnetic vector.

5)Define:
Lp = length of one average charge path poloidal turn
Np = number of charge path poloidal turns
Lt = length of one charge path toroidal turn
Nt = number of charge path toroidal turns.
Rs = toroid outside radius on the equitorial plane
Rc = toroid inside radius on the equitorial plane.

SPHEROMAK CURRENT PATH LENGTH Lh:
Electromagnetic spheromaks arise from the electric current formed by distributed net charge Qs circulating at the speed of light C around the closed spiral path of length Lh which defines the spheromak wall. On the equatorial plane measured from the main axis of symmetry the spheromak inside radius is Rc and the spheromak outside radius is Rs.

Let Np be the integer number of poloidal currrent path turns in Lh and let Nt be the integer number of toroidal current path turns in Lh.

The spheromak wall contains Nt quasi-toroidal turns equally spaced around 2 Pi radians in angle Theta about the main spheromak axis of symmetry.
Each purely toroidal winding turn has length:
2 Pi (Rs - Rc) Kc / 2 = Pi (Rs - Rc) Kc
so the purely toroidal spheromak winding length is:
Nt Pi (Rs - Rc) Kc

Note that for a round spheromak cross section toroid Kc = 1. If for an elliptical cross section spheromak:
[A / B] > 1
then:
Kc > 1

16) In a stable spheromak of a particular size the circulating current:
Ih = Qs Fh = C / Lh
is constant;

17) The circulating current Ih causes a purely toroidal magnetic field inside the spheromak wall and a purely poloidal magnetic field outside the spheromak wall;

18) The net charge Qs causes an electric field outside the spheromak wall which is normal to the spheromak wall in the near field and is spherically radial in the far field;

19) At the center of the spheromak at (R = 0, Z = 0) the net electric field is zero;

20) In the region enclosed by the spheromak wall where:
Rc < R < Rs and |Z| < |Zs|
the total field energy density U takes the form:
Uim = Uio (Ro / R)^2
where:
Uim = toroidal magnetic field energy density inside the spheromak wall;

21) Outside the spheromak wall the total field energy density takes the form:
U = Ue + Um
= Uo [Ro^2 / (Ro^2 + (A R)^2 + (B Z)^2)]^2
where:
Uo = [(Muo Qs^2 C^2) / (32 Pi^2 Ro^4)];

22) Note that this value of Uo comes from integration over a sphere, where:
A > 1
and
B < 1 .

23) Everywhere on the thin spheromak wall:
U = Ueo + Umo = Uei + Umi
where:

Uei = 0

24) In a electromagnetic spheromak the static electric and magnetic field energy density functions are a result of distributed circulating charge that causes the static electric field and that circulates within the spheromak wall with a characteristic frequency:
Fh = C / Lh
causing the static magnetic fields. The charge circulation pattern is described by five parameters: Np, Nt, So, A, B and Ro where:
Np = number of poloidal turns per charge circulation cycle;
Nt = number of toroidal turns per charge circulation cycle;
So^2 = (Rs / Rc) = spheromak shape parameter
Rs = maximum radius from spheromak symmetry axis to spheromak wall;
Rc = minimum radius from spheromak symmetry axis to spheromak wall;
Ro^2 = (A^2 Rs Rc) where Ro is the nominal spheromak radius.
A = [2 Zf / (Rs - Rc)]
B = 1.000
 

SOLUTION FAMILY B:
[Nt / Np] = (P - 2 Np) / Np or
P = 2 Np + Nt
which for:
Np = (Nt + 1)
P = 2(Nt + 1) + Nt
= 3 Nt + 2
For this case:
2 dNp + dNt = 0
Note that in FAMILY B Nt is odd so that P can be odd. Np changes in single steps while Np changes in double steps. Hence only FAMILY B gives a real solution.
Np = Nt + 1  

***************************************************************

SPHEROMAK WALL POSITION:
A spheromak is a stable energy state. The spheromak wall positions itself to achieve a total energy relative minimum consistent with the spheromaks natural frequency Fh. At every point on the spheromak wall the sum of the electric and magnetic field energy densities on the outside side of the spheromak wall equals the toroidal magnetic field energy density on the inside of the spheromak wall. This general statement resolves into different detailed boundary conditions at different points on the spheromak wall. This general boundary condition establishes the spheromak core radius Rc on the equatorial plane, the spheromak outside radius Rs on the equatorial plane and the spheromak length 2 Zf.
 

ENERGY DENSITY BALANCE AT THE SPHEROMAK WALL
Inside the spheromak wall the magnetic field energy density is given by:
Umi = Umic [(Rc / R)^2]

At all points on the spheromak wall the total inside energy density equals the total outside energy density. Hence at all points on the spheromak wall:
Umo + Ueo = Umi

For a spheromak wall position to be stable the total field energy density must be the same on both sides of a thin spheromak wall. This requirement leads to boundary condition equations that determine the shape of a spheromak.

In general the total field energy density U at any point in a spheromak is given by:
U = [Bp^2 / 2 Mu] + [Bt^2 / 2 Mu] + [(Epsilon / 2) Er^2]
where:
Bp = poloidal magnetic field strength;
Bt = toroidal magnetic field strength;
Er = radial electric field strength.

Thus at a spheromak wall:
{[Bp^2 / 2 Mu] + [Bt^2 / 2 Mu] + [(Epsilon / 2) Er^2]}inside
= {[Bp^2 / 2 Mu] + [Bt^2 / 2 Mu] + [(Epsilon / 2) Er^2]}outside
 

However, inside the spheromak wall:
Bp = 0
and
Er = 0
and outside the spheromak wall:
Bt = 0.

Hence at every point on a spheromak wall:
{[[Bt^2 / 2 Mu]}inside
= {[Bp^2 / 2 Mu] + [(Epsilon / 2) Er^2]}outside

At R = Rc and Z = 0:
Symmetry gives:
Eroc = 0
so that:
Umoc = (Umic).
 

APPLICATION OF BOUNDARY CONDITIONS:
1) The energy density in the far field points to Uo in terms of Epsilono.

2) Knowledge of Uo enables calculation of Bpo.

3 Knowledge of Bpo enables calculation of an estimate of Np by assuming that the poloidal current is concentrated in a ring at Z = 0, R = Ro.

4) The boundary condition at the spheromak inner wall enables calculation of Btc in terms of So and hence Bto in terms of So.

5) Bto enables calculation of Nt as a function of So.

6) For a particular So value we can integrate to find the Np value that will yield the required Bpo value.

7) Thus we can find (Np).

8) FIX

9) Knowing the values of Np and (Np / Nt) we can find a consistent prime number Nt.
 

******************************************************************

***********************************************************************

**************************************************************

Assume that the spheromak wall is composed of a current path of length Lh containing uniformly distributed charge Qs that is circulating around a closed spiral path at the speed of light C. The spheromak inside radius measured from the axis of symmetry is Rc and the spheromak outside radius measured from the axis of symmetry is Rs.

In one spheromak cycle the poloidal angle advances Np (2 Pi) radians. In the same cycle the toroidal angle advances Nt (2 Pi) radians. Hence:
(poloidal angle advance) / (toroidal angle advance) = Np / Nt While a current point moves from Rc to Rs the toroidal angle advance is Pi radians and the toroidal travel is Lt / 2. The corresponding distance along the equatorial outer circumference is:
Pi (Np / Nt) Rs.
Thus Pythagoras theorm gives the current point travel distance along the winding for the half toroidal turn as:
[(Lt / 2)^2 + ( Pi Np Rs / Nt)^2]^0.5

Thus the total winding length Lh is:
Lh = Nt [(Lt / 2)^2 + (Pi Np Rs / Nt)^2]^0.5
+ Nt [(Lt / 2)^2 + ( Pi Np Rc / Nt)^2]^0.5
 
= [(Nt Lt / 2)^2 + (Pi Np Rs)^2]^0.5
+ [(Nt Lt / 2)^2 + (Pi Np Rc)^2]^0.5

Recall that:
Lt = [Kc Pi (Rs - Rc)]
and
Rs = Ro So / A
and
Rc = Ro / A So

Thus:
Lh = {(Nt Lt / 2)^2 + (Pi Np Rs)^2}^0.5
+ {(Nt Lt / 2)^2 + (Pi Np Rc)^2}^0.5
 
= {(Nt [Kc Pi (Rs - Rc)] / 2)^2 + (Pi Np Rs)^2}^0.5
+ {(Nt [Kc Pi (Rs - Rc)] / 2)^2 + (Pi Np Rc)^2}^0.5
 
= {[Nt Kc Pi (Rs - Rc) / 2]^2 + (Pi Np Rs)^2}^0.5
+ {[Nt Kc Pi (Rs - Rc) / 2]^2 + (Pi Np Rc)^2}^0.5
 
= {[Nt Kc Pi ((Ro So / 2 A) - (Ro / 2 A So))]^2 + (Pi Np (Ro So / A))^2}^0.5
+ {[Nt Kc Pi ((Ro So / 2 A) - (Ro / 2 A So))]^2 + (Pi Np (Ro / A So))^2}^0.5
 
= [Pi Ro / A]{[Nt Kc ((So / 2) - (1 / 2 So))]^2 + [Np So]^2}^0.5
+[Pi Ro / A] {[Nt Kc ((So / 2) - (1 / 2 So))]^2 + [(Np / So)]^2}^0.5
 
= [Pi Ro / 2 So A] {[Nt Kc ((So^2) - (1))]^2 + [Np (2 So^2)]^2}^0.5
+[Pi Ro / 2 So A] {[Nt Kc ((So^2) - (1))]^2 + [2 Np]^2}^0.5
 
= [Pi Ro / 2 So A] {[Nt Kc (So^2 - 1)]^2 + [Np (2 So^2)]^2}^0.5
+[Pi Ro / 2 So A] {[Nt Kc (So^2 - 1)]^2 + [2 Np]^2}^0.5
 
= [Pi Ro Nt / 2 So A] {[Kc (So^2 - 1)]^2 + [(Np / Nt) (2 So^2)]^2}^0.5
+[Pi Ro Nt / 2 So A] {[Kc (So^2 - 1)]^2 + ([Np / Nt][2])^2}^0.5

Thus:
[Lh A / 2 Pi Ro] = [Nt / 4 So] {[Kc (So^2 - 1)]^2 + (Np / Nt)^2 [2 So^2]^2}^0.5
+ [Nt / 4 So]{[Kc (So^2 - 1)]^2 + (Np / Nt)^2 [2]^2}^0.5
or
[Lh A / 2 Pi Ro] = Nt {[Kc (So^2 - 1) / 4 So]^2 + (Np / Nt)^2 [So / 2]^2}^0.5
+ Nt {[Kc (So^2 - 1)/ 4 So]^2 + (Np / Nt)^2 [(1 / 2 So)]^2}^0.5

This equation is the result of spheromak geometric analysis.

Thus for every So^2 and Kc value there is a corresponding (Nt / Np) value.
Nt = P - 2 Np

Thus for each value of So^2 and Kc and trial P increment through Np to find an approximate zero solution.

The correct value of P will give an exact zero solution.

Note that Np and Nt are positive integers. The quantity [(Lh A / (2 Pi Ro)] is believed to be a geometric constant for a stable spheromak. The stability of this quantity relies on the stabilities of Np, Nt and So.

Note that:
Np = Nt + 1

Note that with increasing spheromak energy Ro and Lh both decrease so that the ratio:
(Lh A / Ro)
remains constant. As Lh decreases the spheromak frequency:
Fh = C / Lh
increases.

The circulating current Ih is given by:
Ih = Qs Fh
= Qs C / Lh where:
Ih = Qs Fh = Qs C / Lh
This equation imposes an important relationship between Nr and So in a spheromak.
 

NATURAL FREQUENCY:
The natural frequency Fh of a spheromak is:
Fh = C / Lh

Note that this formula applies to all spheromaks

*****************************************************************

**************************************************************************

*******************************************************************

****************************************************************

*******************************************************************

AXIAL MAGNETIC FIELD AT CENTER OF A SPHEROMAK:
The poloidal magnetic field Bpo at the center of a spheromak is key to electromagnetic spheromak calculations. It is precisely derived below for both an ellipsoidal cross section spheromak and a round cross section spheromak.

Define:
X = R / Ro
Xs = Rs / Ro = (So / A)
Xc = Rc / Ro = (1 / (A So))

For each specified A and So pair the integral finds the corresponding value of Bpo.

Then:
Bpo = Integral from X = Xc to X = Xs of:
(1 / Ro){(Muo Ih X^2) / {X^2 + (A / B)^2 [Xs - X) (X - Xc)]}^1.5}
{Np / [Pi Kc (Xs - Xc)]} dX
{[(Xs - X) (X - Xc)] + (A / 2 B)^2 [Xs + Xc - 2 X]^2}^0.5 / [(Xs - X)(X - Xc)]^0.5
&n

**********************************************************

************************************************************

****************************************************************

**********************************************************************

MAGNETIC FLUX THROUGH THE CORE OF A SPHEROMAK:
On the Z = 0 plane for 0 < R < Rc:
U = Uo {Ro^2 / [Ro^2 + (A R)^2]}^2
= Uo {A^2 Rs Rc / [A^2 Rs Rc + (A R)^2]}^2
= Uo {Rs Rc / [Rs Rc + (R)^2]}^2

Uo = Bpo^2 / (2 Muo)
U = Bp^2 /(2 Muo)

Thus:
[Bp^2 / 2 Muo] = [Bpo^2 / 2 Muo]{Rs Rc / [Rs Rc + (R)^2]}^2
or
Bp = Bpo {Rs Rc / [Rs Rc + (R)^2]}

Thus the magnetic flux through the spheromak core is:
Integral from R = 0 to R = Rc of:
Bp 2 Pi R dR
 
= Integral from R = 0 to R = Rc of:
Bpo {Rs Rc / [Rs Rc + (R)^2]} 2 Pi R dR

Let X = [Rs Rc + R^2]
dX = 2 R dR
Then the magnetic flux through spheromak core is:
Integral from X = Rs Rc to X = Rs Rc + Rc^2 of:
Bpo Pi dX / X
 
= Bpo Rs Rc Pi Ln[(Rs Rc + Rc^2) / Rs Rc]
 
= Bpo Rs Rc Pi Ln[(Rs + Rc) / Rs]
 
= Bpo (Pi Ro^2 / A^2) Ln[1 + (1 / So^2)]
 

*********************************************************************

*********************************************************************

*********************************************************

********************************************************************

The characteristic frequency of a spheromak is:
F = C / Lh
= C / (Pi {[Nt (Rs - Rc) Kc]^2 + [Np (Rs + Rc)]^2}^0.5)
= C / (Pi Ro {[Nt (So - (1 / So)) Kc]^2 + [Np (So + (1 / So))]^2}^0.5)
= C So / (Pi Ro {[Nt (So^2 - 1) Kc]^2 + [Np (So^2 + 1)]^2}^0.5)

Efs = upper limit on spheromak field energy

FIELD ENERGY DENSITY INSIDE THE SPHEROMAK WALL:
In the toroidal region inside the spheromak wall where Rc < R < Rs and |Z| < |Zs| the total field energy density is given by:
Ut = Uc (Rc / R)^2

This energy density function arises purely from a toroidal magnetic field.
 

CLASSICAL CHARGED PARTICLE RADIUS Re:(this section is not part of the Planck constant derivation)
The classical expression for particle field only considers the electric field energy and assumes a particle radius of Re. The above expression can be compared to classical electric field energy for an electron given by:
Classical electic field = (1 / 4 Pi Epsilon) Q / R^2
El;ectric field Energy density = (Epsilon / 2)(Electric Field)^2
= (Epsilon / 2) [(1 / 4 Pi Epsilon) Q / R^2]^2
and
Electric Field energy = Integral from R = Re to R = infinity of:
(Epsilon / 2) [(1 / 4 Pi Epsilon) Qs / R^2]^2 4 Pi R^2 dR
= Integral from R = Re to R = infinity of:
[(Qs^2 / 8 Pi Epsilon) / R^2] dR
= [(Qs^2 / 8 Pi Epsilon) / Re]
= [(Qs^2 Mu C^2 / 8 Pi) / Re]
where Re is the classical electron radius.

Equating the two expressions for field energy gives:
[(Qs^2 Muo C^2 / 8 Pi) / Re] = (Muo C^2 / 2)[Qs / 4]^2 [1 / Rs Rc]^0.5
or
[(1 / Pi) / Re] = [1 / 4] [1 / Rs Rc]^0.5
or
Re = (4 / Pi) [Rs Rc]^0.5
= (4 / Pi) Ro

It is shown herein that the ratio of Rs to Rc corresponds to a spheromak energy minimum that sets the Planck constant but the precise reasons why electrons and protons have different mass (rest energy) are uncertain.
 ***************************************************************

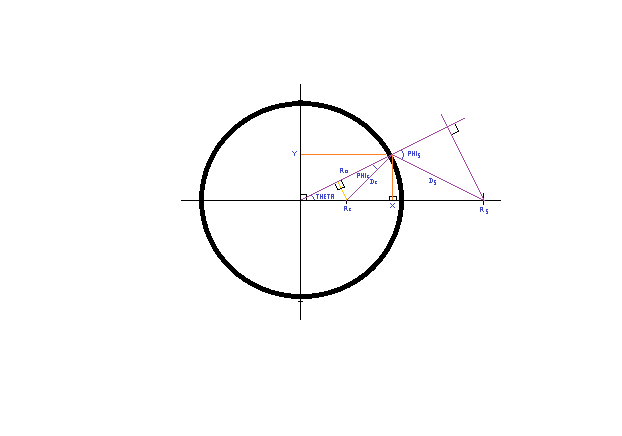
SPHEROMAK WALL BOUNDARY CONDITION AT R = Rc. Z = 0:

Inside the spheromak walls where the static field energy is entirely toroidal magnetic, at R = Rc, Z = 0:
Btc = (Muo Nt Ih / 2 Pi Rc)

The corresponding inside field energy density at R = Rc, Z = 0 is:
Utc = Btc^2 / 2 Muo
= (1 / 2 Muo)[(Muo Nt Ih) / (2 Pi Rc)]^2
= (Muo / 2) [(Nt Ih) / (2 Pi Rc)]^2

Hence the boundary condition at R = Rc, Z = 0 is:
Upc = Utc
or
Uo [Rs / (Rs + Rc)]^2 = (Muo / 2) [(Nt I) / (2 Pi Rc)]^2
or
Uo [Rs / (Rs + Rc)]^2 = (Muo / 2) [(Nt I) / (2 Pi Ro)]^2 [Ro / Rc]^2
or
Uo [So^2 / (So^2 + 1)]^2 = (Muo / 2) [(Nt I) / (2 Pi Ro)]^2 [A So]^2
or
Uo = (Muo / 2) [(Nt I) / (2 Pi Ro)]^2 [A]^2 [(So^2+ 1) / (So)]^2
which is the wall boundary condition at R = Rc, Z = 0.
 

************************************************

**********************************************************

***************************************************

We will test this assumption on the web page titled PLANCK CONSTANT.

*****************************************************

***********************************************************************

MAGNETIC FLUX THROUGH THE CORE OF A SPHEROMAK:
On the Z = 0 plane for 0 < R < Rc:
U = Uo {Ro^2 / [Ro^2 + (A R)^2]}^2
= Uo {A^2 Rs Rc / [A^2 Rs Rc + (A R)^2]}^2
= Uo {Rs Rc / [Rs Rc + (R)^2]}^2

Uo = Bpo^2 / (2 Muo)
U = Bp^2 /(2 Muo)

Thus:
[Bp^2 / 2 Muo] = [Bpo^2 / 2 Muo]{Rs Rc / [Rs Rc + (R)^2]}^2
or
Bp = Bpo {Rs Rc / [Rs Rc + (R)^2]}

Thus the magnetic flux through the spheromak core is:
Integral from R = 0 to R = Rc of:
Bp 2 Pi R dR
 
= Integral from R = 0 to R = Rc of:
Bpo {Rs Rc / [Rs Rc + (R)^2]} 2 Pi R dR

Let X = [Rs Rc + R^2]
dX = 2 R dR
Then the magnetic flux through spheromak core is:
Integral from X = Rs Rc to X = Rs Rc + Rc^2 of:
Bpo Pi dX / X
 
= Bpo Rs Rc Pi Ln[(Rs Rc + Rc^2) / Rs Rc]
 
= Bpo Rs Rc Pi Ln[(Rs + Rc) / Rs]
 
= Bpo (Pi Ro^2 / A^2) Ln[1 + (1 / So^2)]
 

**************************************************************

***************************************************************

ELEMENTAL STRIP ANALYSIS:
Consider an elemental strip of constant radius R. The strip length is:
2 Pi R

The strip width is:
[(Rs - Rc) / 2] dPhi

The strip contains Nt partial windings.

Let L be the length of each partial winding. Then:
L^2 = (R dTheta)^2 + [(Rs - Rc) dPhi / 2]^2

The total charge hose length on the strip is:
Nt L = Nt {(R dTheta)^2 + [(Rs - Rc) dPhi / 2]^2}^0.5
= {(Nt R dTheta)^2 + [(Rs - Rc) Nt dPhi / 2]^2}^0.5
 

SURFACE CHARGE DENSITY:
As shown on the web page titled: THEORETICAL SPHEROMAK the surface charge dQs contained on the elemental strip of constant R is:
dQs = 2 Pi R dLt Sac (Rc / R)
or
Qs = 2 Pi Lt Sac Rc
or
Qs = 2 Pi Lt Sa R
or
Sa = Qs / 2 Pi Lt R

Hence:
dQs = 2 Pi R dLt Sa
= 2 Pi R dLt Qs / 2 Pi Lt R
= dLt Qs / Lt

The corresponding element of area dA is:
dA = 2 Pi R dLt

Hence the charge / unit area Sa on the spheromak wall is:
dQs / dA = (dLt Qs / Lt) / (2 Pi R dLt)
Sa = Qs / {Lt (2 Pi R)]

At R = Rc the charge per unit area Sac is given by:
Sac = Qs / {Lt (2 Pi Rc)]

In general the surface charge density Sa is given by:
Sa = Qs / {Lt (2 Pi R)]

This charge distribution equation is required to find the electric field distribution.
 

POLOIDAL TURNS CONTAINED IN AN ELEMENTAL STRIP:
Recall that:
[dTheta / dPhi] = [Np [(Rs + Rc) / 2] / R Nt]

The number of poloidal turns contained in an elemental strip is:
Nt R dTheta / 2 Pi R = [Nt / 2 Pi] [Np (Rs + Rc) / 2 R Nt] dPhi
= [Np (Rs + Rc) / 4 Pi R] dPhi
where:
[Np (Rs + Rc) / 4 Pi R]
is the number of poloidal turns per radian in Phi
 

*********************************************************************

Note that Ett is a function of Uo, Ro, A and So. Hence in electromagnetic analysis of a spheromak we seek to find expressions for these parameters.

SPHEROMAK STATIC FIELD ENERGY:
The web page titled SPHEROMAK ENERGY shows that the energy density functions:
U = Uo [Ro^2 / (Ro^2 + (A R)^2 + (B Z)^2)]^2
outside a spheromak wall and
U = Uoc [(Rc / R)^2]
inside a spheromak wall result in spheromaks with total energy given by:
Ett = Uo Pi^2 Ro^3/ A^2
X {1 - [(So - 1)^2 / (So^2 + 1)] + [2 So (So - 1)^2 / (So^2 + 1)^2]}
 
= Uo Pi^2 Ro^3 {4 So (So^2 - So + 1) / (So^2 + 1)^2}
Note that this formula applies to all spheromaks.
 

The energy density distribution:
U = Uo [Ro^2 / (Ro^2 + (A R)^2 + Z^2)]^2
provides an energy density of:
U = Uo [Ro^2 / (Ro^2 + (A Rc)^2)]^2
at R = Rc, Z = 0.

SPHEROMAK ENERGY CONTENT:
Substitution for Uo gives:
Efs = Uo Ro^3 Pi^2 / A^2
= (Muo / 2) Ro^3 Pi^2 [(Qs C) /(4 Pi Ro^2)]^2
= (Muo / 32) (Qs C)^2 /(Ro)
and
Ett = [Muo (Qs C)^2 / (32 Ro)] {1 -[(So - 1)^2 / (So^2 + 1)] + [2 So (So - 1)^2 / (So^2 + 1)^2]}
or
Ett = [(Muo C^2 Qs^2) / (32 Ro)]{4 So (So^2 - So + 1) / (So^2 + 1)^2}

This equation gives the static electromagnetic field energy content of a spheromak in terms of its nominal radius Ro and its shape factor So where:
So = Rs / Ro = Ro / Rc

Thus an electromagnetic spheromak has a net charge Qs, a nominal radius Ro and a theoretical peak central poloidal magnetic field strength given by:
Bpo = [(Muo C Qs) / (4 Pi Ro^2)]

This value of Bpo should equal the value of Bpo obtained by applying the law of Biot and Savart to the circulating charge in the spheromak.
 

MAXIMUM ENERGY VALUE OF So:
An important issue is finding the value of So which maximizes a spheromak's energy content at any particular value of Ro. At that energy maximum:
dEtt / dSo = 0

Recall that:
Ett = [(Muo C^2 Qs^2) / (32 Ro)]{4 So (So^2 - So + 1) / (So^2 + 1)^2}

The So dependent portion of the function is:
S(So) = {So (So^2 - So + 1) / (So^2 + 1)^2}

dS(So) / dSo = {(So^2 + 1)^2 [(So^2 - So + 1) + So (2 So - 1)]
- [So (So^2 - So + 1)] 2 (So^2 + 1) 2 So}
/ (So^2 + 1)^4
= 0

Hence:
{(So^2 + 1)^2 [(So^2 - So + 1) + So (2 So - 1)]
- [So (So^2 - So + 1)] 2 (So^2 + 1) 2 So}
= 0

Cancelling (So^2 + 1) terms gives:
{(So^2 + 1) [(So^2 - So + 1) + So (2 So - 1)]
- [4 So^2 (So^2 - So + 1)]}

Hence:
(So^2 + 1 - 4 So^2)(So^2 - So + 1) + (So^2 + 1) So (2 So - 1) = 0
or
(- 3 So^2 + 1)(So^2 - So + 1) + (So^2 + 1)(2 So^2 - So) = 0
or
- 3 So^4 + 3 So^3 - 3 So^2 + So^2 - So + 1 + 2 So^4 - So^3 + 2 So^2 - So = 0
or
- So^4 + 2 So^3 - 2 So + 1 = 0
or
So^4 - 2 So^2 + 2 So - 1 = 0
or
So^4 - So^2 = So^2 - 2 So + 1
or
So^2 (So^2 - 1) = (So - 1)^2
or
So^2 = (So - 1)^2 / [(So - 1) (So + 1)]
= (So - 1) / (So + 1)

This equation has a solution of So = 1 which says that at a particular Ro the spheromak's energy is maximum at So = 1, at which point Rc = Ro = Rs corresponding to no volume inside the spheromak wall.

Thus at the maximum energy state: {So (So^2 - So + 1) / (So^2 + 1)^2}
= {1 (1 - 1 + 1) / (1 + 1)^2}
= 1 / 4

At So = 2:
(spheromak energy)
= {So (So^2 - So + 1) / (So^2 + 1)^2}
= {2 (4 - 2 + 1) / (4 + 1)^2}
= {6 / 25}
= which is only slightly less than the spheromak maximum energy.

Thus for modest So values of the order of So < 2 the static field energy content of a spheromak is only weakly dependent on the spheromak's So value. However, at larger So values the spheromak static field energy is proportional to (1 / So).
 

**************************************************************************** *******************************************************************************<

MERGING AXIAL ELECTRIC FIELD AND MAGNETIC FIELDS OF A SPHEROMAK

Hence the Fine Structure constant definitely arises from the spheromak relationship and the relative strength of electric and magnetic fields. We need a more exact solution to properly evaluate it.

At R = Rs, Z = 0:
Toroidal magnetic field must approximately match the increase in the electric field. The increase in electric field energy density is given by:
(Epsilono / 2)(Sac Rc / Epsilono Rs)^2

The drop in toroidal magnetic field energy density is:
(1 / 2 Muo)(Muo Nt Q Fh / 2 Pi Rs)^2
= (1 / 2 Muo)(Muo Nt Q C / 2 Pi Rs Lh)^2

Thus, equating these two expressions gives:
(1 / 2 Epsilono)(Sac / So^2)^2 = (Muo / 2)(Nt Q C / 2 Pi Ro So Lh)^2
or
(Sac / So)^2 = (Epsolono Muo) (Nt Q C / 2 Pi Ro Lh)^2
= (Nt Q / 2 Pi Ro Lh)^2
or
(Sac / So) = (Nt Q / 2 Pi Ro Lh)
or
Sac = (Nt Q So / 2 Pi Ro Lh)

Recall that the spheromak surface charge distribution is given by:
Sac = [Qs So^2] / [2 Pi^2 Ro^2 ( So^2 - 1)]WRONG

Equating the two expressions for Sac gives:
(Nt Q So / 2 Pi Ro Lh) = [Qs So^2] / [2 Pi^2 Ro^2 ( So^2 - 1)]
or
(Nt / Lh) = So / [Pi Ro (So^2 - 1)]
or
(Lh / Ro) = Pi (So^2 - 1) Nt / So

This is a tremendously revealing result. It is part of:
(Lh / Ro) = (Pi / So)[Np^2 (So^2 + 1)^2 + Nt^2 (So^2 - 1)^2]^0.5

Note that the poloidal and toroidal magnetic fields are orthogonal.

Hence at R = Rs the poloidal magnetic field energy density is given by:
(Epsilono / 2)[Sac Rc / Epsilono Rs]^2 = Bps^2 / 2 Muo
FIX where:
Bps = Muo Np Qs Fh / 2 Pi Rx
= (Bpc Rc / Rs)^2 / 2 Muo
due to the change in perimeter lengths.

The change in radial electric field at R = Rc, H = 0 causes an energy density balance at R = Rc of:
[(Bpc)^2 / 2 Muo] - [(Btc^2 / 2 Muo] = (Epsilono / 2)[Sac / Epsilono]^2
or
[(Bpc)^2 / 2 Muo] = [(Btc^2 / 2 Muo] + (Epsilono / 2)[Sac / Epsilono]^2

If the spheromak was a straight core the poloidal magnetic field at the spheromak wall would be:
Muo Np Q Fh / [2 Pi (Rs - Rc) / 2]

The thesis is that the total energy contained in a spheromak is:
Outside Electric field energy + Outside Poloidal Magnetic field energy + Toroidal magnetic field energy.

From the web page titled: SPHEROMAK ENERGY:
Toroidal magnetic field energy + internal electric field energy
= Poloidal magnetic field energy + external electric field energy = ????????????????

THE INDEX N:
On this web page the total field energy density U is expressed as:
U = Ue + Um
where Ue is the electric field energy density component and Um is the magnetic field energy density component.

Note that Uoc reflects the reality that R = Rc and Z = 0 there is a radial electric field Eroc pointing toward the spheromak axis of symmetry.

Note that at R = 0, Z = 0:
Umo = Uo

Note that in general outside the spheromak wall:
U = Uo [Ro^2 / (Ro^2 + A^2 R^2 + Z^2)]^2 which is the equation for the total field energy density outside a spheromak wall.

The expressions for Ue and Um in the far field match the well known far field dependences of electric and magnetic field energy densities on distance.
 

STRATEGY:
Find the electric and magnetic field energy densities along the spheromak main axis of symmetry. Compare this function to:
U = Uo [Ro^2 / (Ro^2 + Z^2)]^2 and try to determine A via its effect on the electric and magnetic field energy densities along the Z axis.
 

*************************************************************** ***************************************************************

SPHEROMAK ENERGY RATIO:
An issue in electromagnetic spheromaks is the ratio of electric field energy to total energy. On the web page titled SPHEROMAK ENERGY it was shown that the total contained electomagnetic energy of a spheromak before adjustment for the toroidal portion is:
Efs = Uo Ro^3 Pi^2
and the spheromak energy Ett after adjustment for the toroidal portion is:
Ett / Efs
= {1 -[(So - 1)^2 / (So^2 + 1)] + [2 So (So - 1)^2 / (So^2 + 1)^2]}

For R > Rc the electric field energy density of a spheromak outside the spheromak wall is given by:
Ueo = Uo [Ro^2 / (Ro^2 + R^2 + H^2)]^2 [(R^2 + H^2) / (Ro^2 + R^2 + H^2)]^N

Let Z^2 = R^2 + H^2

Then:
Ueo = Uo [Ro^2 / (Ro^2 + Z^2)]^2 [(Z^2) / (Ro^2 + Z^2)]^N

The corresponding total electric field energy is given by:
Integral from Z = 0 to Z = infinity of:
Uo 4 Pi Z^2 dZ Ro^4 (Z^2)^(2 N) / (Ro^2 + Z^2)^(N + 2)
 

From Dwight XXXX the solution to this integral is:
DO INTEGRATION

The corresponding total magnetic field energy is given by:
Integral from Z = 0 to Z = infinity of:
Uo 4 Pi Z^2 dZ Ro^4 [(Ro^2 + Z^2)^N - (Z^2)^N] / (Ro^2 + Z^2)^(N + 2)
 

From Dwight XXXX the solution to this integral is:
DO INTEGRATION

Thus the electric field energy contained in Efs is about ____% of the total field energy of Efs and the magnetic field energy is about ______% of the total field energy of Efs.
 

******************************************************************************* *************************************************************************

SPHEROMAK WALL THEORY REVIEW:
On the web page titled CHARGE HOSE PROPERTIES it was shown that for a charge hose (charge motion path) current Ih is given by:
Ih = (1 / Lh) [Qp Nph Vp + Qn Nnh Vn]
= Qs C / Lh
and
Rhoh = (1 / Lh) (Qp Nph + Qn Nnh)
= Qs / Lh
giving:
(Ih / C)^2 = Rhoh^2
= (Qs / Lh)^2
= (1 / Lh)^2 [Qp Nph + Qn Nnh]^2
and that for practical ionized gas plasmas where Ve^2 >> Vi^2 and Ne ~ Ni:
Ih^2 ~ [Q Ne Ve / Lh]^2
and
[(Ni - Ne) / Ne]^2 ~ (Ve / C)^2
and
Qs^2 ~ [Q Ne Ve / C]^2

These equations allow the development of electromagnetic spheromak theory for atomic particles and plasma.
 

******************************************************************** ********************************************************************************

CONNECTING SPHEROMAK THEORY TO THE FINE STRUCTURE CONSTANT:

The total spheromak energy E is of the form:
E = R(Ro) S(So) where:
R(Ro) is a function proportional to (1 / Ro) and hence to natural frequency Fh and S(So) is a function consisting of two orthogonal terms, one proportional to the number of spiral path poloidal turns Np and the other proportional to the number of spiral path toroidal turns Nt.

Existence and boundary conditions impose a mathematical relationship between these two orthogonal energy terms.
 

Thus we can readily compute the corresponding [Z^2 / Nt^2] value. We can use this methodology to find [Z / Nt] as a function of So and hence (1 / (Alpha Nt) as a function of So.

We can find the So value corresponding to the stable So value in a plot of (1 / Alpha Nt)^2 versus So. The Alpha value at this point is the Fine Structure constant. However, to determine Alpha we must first determine (1 / Alpha Nt) an

Then we recognize that in a spheromak:
Nr = Np / Nt
where Np and Nt are integers with no common factors.
 

Recall that:
Nr^2 = Np^2 / Nt^2
where Np and Nt are integers with no common factors. Thus to find Np and Nt it is necessary to test both the Np and Nt values for integer and factor compliance. This is not a huge task because we know that:
(1 / Alpha) ~ 137
constrains the maximum size of the Np and Nt integer values to less than about 500.

We know that with the simple boundary condition:
(1 / Alpha Nt)^2 = (Pi / 4)^2 [(So^2 - So + 1)^2 / (So^2 + 1)^8] {2 (So^2 + 1)^2 - 4}
/ {- [4 / [(So^2 - 1)^2]] + [Pi^2 / 4]}

We know that:
(1 / Alpha) ~ 137

Hence we can estimate Nt using the equation:
Nt|estimate = (1 / Alpha) / (1 / (Alpha Nt))
= 137 /(1 / (Alpha Nt))

Then we can estimate Np using the equation:
Np|estimate = Nr Nt|estimate
where to calculate Nr we first calculate:
[Z^2 / Nt^2] = {2 - [4 / (So^2 + 1)^2]}
/ {+ [Pi^2 / 4][1 / (So^2 + 1)^2] - [4 / [(So^2 + 1)^2 (So^2 - 1)^2]]} and then calculate Nr^2 using the equation:
Nr^2 = {Z^2 / [Nt^2 (So^2 + 1)^2]} - {[(So^2 - 1)^2 / (So^2 + 1)^2]}
and the calculate Nr using the equation: Nr = [Nr^2]^0.5

These estimates in combination with a list of prime numbers lead to only a few Np, Nt combinations that need to be fully tested.

Nr = Np / Nt
where Np and Nt are both integers with no common factors and usually either Np or Nt is prime.
 

Based on experience with plasmas I expected that the operating So^2 value would be:
So^2 ~ 4.1.

Moving the operating So value requires a change in the electric field energy distribution function. This issue needs more study.

Calculate the corresponding [Z^2 / Nt^2] value.

Calculate the corresponding value of Nr^2 using the formula:

Calculate the exact value of Nr using the formula:
Nr = [Nr^2]^0.5

Find Np and Nt which are the smallest integers with no common factors that precisely satisfy the equation:
Nr = (Np / Nt)

Usually Np and Nt have no common factors because one of them is prime.

Calculate the error in Nr base on Np = 223 and Nt = 303.

It is the precision of this ratio of integers coincident with real numbers which are a function of Pi that causes spheromak stability and hence quantization of energy.

Once Nt is precisely determined use the formula:
[1 / Alpha] = [Nt] [1 / (Alpha Nt)]
to determine the calculated value of:
[1 / Alpha].

Check if the calculated value of [1 / Alpha] is close to the value:
(1 / Alpha) = 137.035999
which is published at:
Fine Structure Constant

***********************************************************

SUMMARY:
An electromagnetic spheromak is governed by an existence condition and a common boundary condition. After an electromagnetic spheromak forms it spontaneously emits photons until it reaches an energy minimum also known as a ground state .

SPHEROMAK SHAPE PARAMETER:
See the new web page titled: SPHEROMAK SHAPE PARAMETER.
 

SPHEROMAK EVOLUTION:
So^2 = (Rs / Rc) = 4.2
obtained from a General Fusion plasma spheromak photograph. However, a plasma spheromak may be affected by inertial forces and other issues that do not affect a charged particle spheromak.
 

************************************************************************

PLASMA SPHEROMAKS:

SPHEROMAK WALL THEORY REVIEW:
On the web page titled CHARGE HOSE PROPERTIES it was shown that for a charge hose (charge motion path) current Ih is given by:
Ih = (1 / Lh) [Qp Nph Vp + Qn Nnh Vn]
= Qs C / Lh
and
Rhoh = (1 / Lh) (Qp Nph + Qn Nnh)
= Qs / Lh
giving:
(Ih / C)^2 = Rhoh^2
= (Qs / Lh)^2
= (1 / Lh)^2 [Qp Nph + Qn Nnh]^2
and that for practical ionized gas plasmas where Ve^2 >> Vi^2 and Ne ~ Ni:
Ih^2 ~ [Q Ne Ve / Lh]^2
and
[(Ni - Ne) / Ne]^2 ~ (Ve / C)^2
and
Qs^2 ~ [Q Ne Ve / C]^2

These equations allow the development of electromagnetic spheromak theory for atomic particles and plasma.
 

Define:
Ih = plasma hose current
C = speed of light
Rhoh = (Qs / Lh)

Recall from PLASMA HOSE THEORY that:
(Ih / C) = Rhoh
= (Qs / Lh)
= Qs / [(Np Lp)^2 + (Nt Lt)^2]^0.5
or
Ih = C Qs / [(Np Lpf)^2 +(Nt Lt)^2]^0.5
= Qs C / {Pi Ro [[(Np (Rs + Rc))^2 / (Ro)^2] + [(Nt (Rs - Rc))^2 / (Ro)^2]]^0.5}
Thus if the charge Q on an atomic particle spheromak is replaced by the net charge Qs on a plasma spheromak the form of the spheromak equations is identical.

However, in a plasma spheromak:
Qs = Q (Ni - Ne)
where (Ni - Ne) is positive.

The web page PLASMA HOSE THEORY shows that for a plasma spheromak:
(Ni - Ne)^2 C^2 = (Ne Ve)^2
where Ve = electron velocity.

The kinetic energy Eke of a free electron with mass Me is given by:
Eke = (Me / 2) Ve^2

Hence:
(Ni - Ne)^2 C^2 = Ne^2 (2 Eke / Me)
or
Qs = Q (Ni - Ne) = Q (Ne / C)[2 Eke / Me]^0.5

Thus in a plasma spheromak Qs can potentially be obtained via measurements of Ne and Eke. However, due to the free electrons being confined to the spheromak wall Ne is not easy to accurately directly measure.
 

CALCULATION OF PLASMA SPHEROMAK Ne FROM THE FAR FIELD:
An approximate expression for the distant radial electric field is:
[Qs / 4 Pi Epsilon] [1 / (R^2 + H^2)]

The corresponding far field energy density is:
Ue = (Epsilon / 2)[Qs / (4 Pi Epsilon)]^2 [1 / (R^2 + H^2)]^2
= [Qs^2 / (32 Pi^2 Epsilon)][1 / (R^2 + H^2)]^2

***********************************************************

FIX THE A and Z TERMS FROM HERE ONWARDS:
and that this energy density in the far field must equal
Uo [Ro^2 / (Ro^2 + R^2 + H^2)]^2
where:
Uo = (Bpo^2 / 2 Mu)
Equating the two energy density expressions in the far field gives:
[Qs^2 / (32 Pi^2 Epsilon)] [1 / (R^2 + H^2)]^2
= (Bpo^2 / 2 Mu) [Ro^2 / (Ro^2 + R^2 + H^2)]^2
or
[Qs^2 / (32 Pi^2 Epsilon)] = (Bpo^2 / 2 Mu) [Ro^2]^2
or
Qs^2 = (Bpo^2 / 2 Mu) [Ro^2]^2 (32 Pi^2 Epsilon)
= (Bpo^2 / Mu) [Ro^2]^2 (16 Pi^2 / C^2 Mu)
= (Bpo^2 / Mu^2) [Ro^2]^2 (16 Pi^2 / C^2)

Thus:
Qs = (Bpo / Mu) [Ro^2] (4 Pi / C)

Recall that the formula for a plasma spheromak gave:
Qs = Q (Ne / C)[2 Eke / Me]^0.5
or
Ne = Qs C /(Q [2 Eke / Me]^0.5)
= (Bpo / Mu) [Ro^2] (4 Pi / C) C /(Q [2 Eke / Me]^0.5)
= (Bpo / Mu) [Ro^2] (4 Pi /(Q [2 Eke / Me]^0.5)
= (Bpo / Mu) [Rs Rc] (4 Pi /(Q [2 Eke / Me]^0.5)

This equation can be used to estimate Ne in experimental plasma spheromaks.
 

For a spheromak compressed from state a to state b this equation can be written in ratio form as:
(Neb / Nea) = (Bpob / Bpoa)(Rsb Rcb / Rsa Rca) (Ekea / Ekeb)^0.5
or
(Neb / Nea)^2 = (Bpob / Bpoa)^2(Rsb Rcb / Rsa Rca)^2 (Ekea / Ekeb)
or
(Neb / Nea)^2(Roa^6 / Rob^6)
= (Bpob / Bpoa)^2(Rsb Rcb / Rsa Rca)^2 (Roa^6 / Rob^6)(Ekea / Ekeb)
= (Bpob / Bpoa)^2(Rsb Rcb / Rsa Rca)^2 [(Rsa Rca)^3 / (Rsb Rcb)^3] (Ekea / Ekeb)
= (Bpob / Bpoa)^2 [(Rsa Rca) / (Rsb Rcb)] (Ekea / Ekeb)
 

EXPERIMENTAL PLASMA SPHEROMAK DATA:
General Fusion has reported spheromak free electron kinetic energies ranging from 20 eV - 25 eV for low energy density spheromaks at the spheromak generator to 400 ev - 500 eV for higher energy density spheromaks at the downstream end of the conical plasma injector. General Fusion reports a spheromak linear size reduction between these two positions of between 4X and 5X. The corresponding observed apparent electron densities rise from 2 X 10^14 cm^-3 to 2 X 10^16 cm^-3. The corresponding observed magnetic field increases from .12 T to 2.4 T to 3 T. At this time this author does not know for certain: where on the spheromak the electron kinetic energy was measured, where on the spheromak the apparent electron density was measured, where on the spheromak the magnetic field was measured or the absolute dimensions of the measured spheromaks and their enclosure.

Hence:
16 < [Ekeb / Ekea] < 25
20 < (Bpob / Bpoa) < 25
400 < (Bpob / Bpoa)^2 < 625<
4 < (Rca / Rcb) < 5
16 < (Rca / Rcb)^2 < 25
64 < (Rca / Rcb)^3 < 125
[(Nea / Rca^3) / (Neb / Rcb^3)]^2 = 10^-2

It appears that during the plasma spheromak compression Nea decreases to Neb while Qs remains constant. This effect might be due to electron-ion recombination during spheromak compresion.
 

DISTANCE BETWEEN ADJACENT CURRENT FILAMENTS:
At R = Rc, Z = 0 the distance between adjacent filaments measured in the equatorial plane is:
2 Pi Rc / Nto = dPhi Rc
or:
dPhi = 2 Pi / Nto

At R = Rc, Z = 0 the distance between adjacent filaments measured perpendicular to the equatorial plane is:
[(Ro - Rc)] dTheta = [Ro - Rc)][(dTheta / dPhi)|Rc] dPhi = ((Ro - Rc))[(dTheta / dPhi)|Rc](2 Pi / Nto)
= (Rs - Rc) Pi [(dTheta / dPhi)|Rc] / Nto

At R = Ro , Z = H the distance between adjacent filaments measured parallel to the minor axis is:
2 Pi [Ro] / Nto = [Ro] dPhi
or: dPhi = 2 Pi / Nto

At R = Ro, Z = H the distance between adjacent filaments measured along a radial line is:
H dTheta = H [(dTheta / dPhi)|Ro] dPhi
= H [(dTheta / dPhi)|Ro] (2 Pi / Nto)

At R = Rs, Z = 0 the distance between adjacent filaments measured along the equatorial plane is:
Rs dPhi = 2 Pi Rs / Nto
or
dPhi = 2 Pi / Nto

At R = Rs, Z = 0 the distance between filaments measured parallel to the Z axis is:
[(Rs- Rc) / 2] dTheta = [(Rs - Rc) / 2] [(dTheta / dPhi)|Rs] dPhi
= [(Rs - Rc) / 2][(dTheta / dPhi)|Rs][2 Pi / Nto]
= [(Rs - Rc)][(dTheta / dPhi)|Rs][Pi / Nto]

The above calculated distances can be used to compute the shortest separation between adjacent filaments at each of the three specified locations. The (shortest separation) X (Q / Lh) = charge / unit area The surface electric field Es = (charge / unit area) / Epsilono
 

SHORTEST DISTANCE BETWEEN ADJACENT FILAMENTS
Let Dh = horizontal distance between adjacent filaments
Let Dv = vertical distance between adjacent filaments
Let X = shortest distance betwen adjacent filaments.

Then a little geometry shows that:
Dh^2 + Dv^2 = {[Dh^2 - X^2]^0.5 + [Dv^2 - X^2]^0.5}^2
or
Dh^2 + Dv^2 = Dh^2 - X^2 + Dv^2 - X^2 + 2{[Dh^2 - X^2]^0.5 [Dv^2 - X^2]^0.5}
or
2 X^2 = 2{[Dh^2 - X^2]^0.5 [Dv^2 - X^2]^0.5}
or
X^4 = [Dh^2 - X^2] [Dv^2 - X^2]
or
X^4 = Dh^2 Dv^2 + X^4 - X^2 (Dv^2 + Dh^2)
or
X^2 = Dh^2 Dv^2 / (Dv^2 + Dh^2)
or
X = Dh Dv / (Dv^2 + Dh^2)^0.5
 

SHORTEST DISTANCE BETWEEN ADJACENT FILAMENTS AT R = Rc, Z = 0:
Dh = 2 Pi Rc / Nto
Dv = (Rs - Rc) Pi [(dTheta / dPhi)|Rc] / Nto
Dh Dv = 2 Pi^2 Rc (Rs - Rc) [(dTheta / dPhi)|Rc] / Nto^2
Dh^2 = 4 Pi^2 Rc^2 / Nto^2
Dv^2 = (Rs - Rc) Pi^2 [(dTheta / dPhi)|Rc]^2 / Nto^2
Xc = Dh Dv / (Dv^2 + Dh^2)^0.5>
= [2 Pi^2 Rc (Rs - Rc)[(dTheta / dPhi)|Rc] / Nto^2] / [(4 Pi^2 Rc^2 / Nto^2) + ((Rs - Rc)^2 Pi^2 [(dTheta / dPhi)|Rc]^2 / Nto^2)]^0.5
= [2 Pi Rc (Rs - Rc)[(dTheta / dPhi)|Rc] / Nto] /[(4 Rc^2) + ((Rs - Rc)^2[(dTheta / dPhi)|Rc]^2)]^0.5
 

SHORTEST DISTANCE BETWEEN ADJACENT FILAMENTS AT R = (Rs + Rc) / 2, Z = H:
Dh = 2 Pi [(Rs + Rc) / 2] / Nto
Dv = H [(dTheta / dPhi)|Rm] (2 Pi / Nto)
Dh Dv = 2 Pi^2 H [Rs + Rc][(dTheta / dPhi)|Rm] / Nto^2
Dh^2 = Pi^2 (Rs + Rc)^2 / Nto^2
Dv^2 = 4 Pi^2 H^2 [(dTheta / dPhi)|Rm]^2 / Nto^2
Xm = Dh Dv / (Dv^2 + Dh^2)^0.5
= [2 Pi^2 H [Rs + Rc][(dTheta / dPhi)|Rm] / Nto^2] / [(Pi^2 (Rs + Rc)^2 / Nto^2) + (4 Pi^2 H^2 [(dTheta / dPhi)|Rm]^2 / Nto^2)]^0.5
= [2 Pi H [Rs + Rc][(dTheta / dPhi)|Rm]] / [((Rs + Rc)^2) + (4 H^2 [(dTheta / dPhi)|Rm]^2)]^0.5
 

SHORTEST DISTANCE BETWEEN ADJACENT FILAMENTS AT R = Rs, Z =0:
Dh = 2 Pi Rs / Nto
Dv = [(Rs - Rc)][(dTheta / dPhi)|Rs][Pi / Nto]
Dh Dv = 2 Pi^2 Rs (Rs - Rc)[(dTheta / dPhi)|Rs] / Nto^2
Dh^2 = 4 Pi^2 Rs^2 / Nto^2
Dv^2 = Pi^2 (Rs - Rc)^2 [(dTheta / dPhi)|Rs]^2 / Nto^2
Xs = Dh Dv / (Dv^2 + Dh^2)^0.5
= [2 Pi^2 Rs (Rs - Rc)[(dTheta / dPhi)|Rs] / Nto^2] / [(4 Pi^2 Rs^2 / Nto^2) + (Pi^2 (Rs - Rc)^2 [(dTheta / dPhi)|Rs]^2 / Nto^2)]^0.5
= [2 Pi Rs (Rs - Rc)[(dTheta / dPhi)|Rs] / Nto] / [(4 Rs^2) + ((Rs - Rc)^2 (dTheta / dPhi)|Rs]^2)]^0.5
 

SURFACE ELECTRIC FIELD:
The outward pointing surface electric field Es resulting from a surface charge per unit area is:
Es = Q / (Lh X Epsilono)
where:
Lh = [(Npo Lp)^2 + (Nto Lt)^2]^0.5
= [(Npo Pi (Rs + Rc))^2 + (Nto Lt)^2]^0.5
 

INTERIOR ELECTRIC FIELD CANCELATION:
Note that inside the spheromak wall, in order to have no net electric field on the equatorial plane, the radial electric field must be inversely proportional to X. Hence:
Ess / Esc = Rc / Rs

However:
Ess / Esc = [Xc / Xs]
= {[2 Pi Rc (Rs - Rc)[(dTheta / dPhi)|Rc] / Nto] /[(4 Rc^2) + ((Rs - Rc)^2 [(dTheta / dPhi)|Rc]^2)]^0.5}
/ [2 Pi Rs (Rs - Rc)[(dTheta / dPhi)|Rs] / Nto] / [(4 Rs^2) + ((Rs - Rc)^2 [(dTheta / dPhi)|Rs]^2)]^0.5

In order to have no net electric field inside the spheromak wall:
{[[(dTheta / dPhi)|Rc] / Nto] / [(4 Rc^2) + ((Rs - Rc)^2 [(dTheta / dPhi)|Rc]^2)]^0.5}
= [[(dTheta / dPhi)|Rs] / Nto] / [(4 Rs^2) + ((Rs - Rc)^2 [(dTheta / dPhi)|Rs]^2)]^0.5

Try for a general solution to this equation of the form:
[[(dTheta / dPhi)|R] / Nto] / [(4 R^2) + ((Rs - Rc)^2 [(dTheta / dPhi)|R]^2)]^0.5 = K = constant
which is valid for all R values.

Then:
[[(dTheta / dPhi)|R] / Nto]^2
= K^2 [(4 R^2) + ((Rs - Rc)^2 [(dTheta / dPhi)|R]^2)]
or
[(dTheta / dPhi)|R]^2 {(1 / Nto^2) - K^2 (Rs - Rc)^2} = 4 K^2 R^2
or
[(dTheta / dPhi)|R]^2 = 4 K^2 R^2 / {(1 / Nto^2) - K^2 (Rs - Rc)^2}
or
[(dTheta / dPhi)|R]^2 = 4 K^2 R^2 Nto^2 / {1 - Nto^2 K^2 (Rs - Rc)^2}
[(dTheta / dPhi)|R] = 2 K R Nto / {1 - Nto^2 K^2 (Rs - Rc)^2}^0.5

Recall that the average value of (dTheta / dPhi) = (Nto / Npo).
Hence integral from R = Rc to R = Rs of
[(dTheta / dPhi)|R]dR / (Rs - Rc)
= (Nto / Npo) or K (Rs^2 - Rc^2) Nto / {{1 - Nto^2 K^2 (Rs - Rc)^2}^0.5 (Rs - Rc)}
= (Nto / Npo) or
K (Rs + Rc) Nto / {1 - Nto^2 K^2 (Rs - Rc)^2}^0.5 = (Nto / Npo) or
K Npo (Rs + Rc) / {1 - Nto^2 K^2 (Rs - Rc)^2}^0.5 = 1
or
K^2 Npo^2 (Rs + Rc)^2 = 1 - Nto^2 K^2 (Rs - Rc)^2
or
K^2 {Npo^2 (Rs + Rc)^2 + Nto^2 (Rs - Rc)^2} = 1

This is an important equation because it relates constant K to filament length Lh for a spheromak. This equation arose from the requirement that the net radial electric field inside the spheromak wall be zero.
 

ELECTRIC FIELD COMMENTARY:
Ideally the shortest separation between adjacent filament turns on the ellipsoid surface should be proportional to (1 / R). Then the outward pointing electric field Es on the spheromak surface will be proportional to (1 / R). Then, due to cylindrical symmetry, inside the spheromak wall the net electric field will be zero. Outside the spheromak wall the electric field is proportional to the surface charge density.

On the equatorial plane, due to cylindrical symmetry, for R < Rc the net electric field should be zero.

At R = Rc, Z = 0 the electric field inside the spheromak wall due to the inner wall pointing radially outward is proportional to (1 / Rc). when this field reaches the outer wall, due to cylindrical symmetry the electric field strength is proportional to:
(1 / Rc)(Rc / Rs) = (1 / Rs).

At R = Rm = (Rs + Rc) / 2, Z = H the surface electric field points along an outward pointing vector involving both Rm and H and should be proportional to [2 / (Rs + Rc)].

At R = Rs, Z = 0 the surface electric field points radially and should be proportional to (1 / Rs).

To achieve this electric field pattern objective the spacing between adjacent filament turns must change with R as set out above.

At R = Rs, Z = 0 the electric field pointing radially outwards is: Es = 2 (Surface charge / unit area)(1 / Epsilono)
= 2 (Q / Lh)[1 / X](1 / Epsilono)

Note that this electric field is a result of both the surface charge on the outer wall and the surface charge on the inner wall.

Note that in terms of angle about the major axis of symmetry the number of toroidal turn equatorial plane crossings per unit angle is the same for both the inner and the outer wall.

Inside the toroidal region the electric fields parallel to the Z axis emitted by the spheromak ends cancel.
 

It is tempting to attempt to simplify the situation by assuming that a spheromak winding everywhere follows:
dTheta / dPhi = Npo / Nto,
but while this equation is true on average making that assumption at each point on the winding does not lead to a net zero electric field inside the spheromak wall.

Instead, we found that to achieve a net zero electric field inside the spheromak wall:
[(dTheta / dPhi)|R] = 2 K R Nto / {1 - Nto^2 K^2 (Rs - Rc)^2}^0.5
where K is a constant.

After taking into account the required average value of [(dTheta / dPhi)average] = [Nto / Npo]
we found the important spheromak constraint that:
K^2 {Npo^2 (Rs + Rc)^2 + Nto^2 (Rs - Rc)^2} = 1

For a spheromak with a round cross section:
Lh^2 = (Npo Lp)^2 + (Nto Lt)^2
= (Npo 2 Pi (Rs + Rc) / 2)^2 + (Nto 2 Pi (Rs - Rc) / 2)^2
= (Npo Pi (Rs + Rc))^2 + (Nto Pi (Rs - Rc))^2
= Pi^2 (Npo (Rs + Rc))^2 + (Nto (Rs - Rc))^2

Hence:
K = Pi / Lh

Note that for a spheromak with a round cross section K is proportional to the spheromak's frequency and total energy.

At a particular spheromak energy:
(Pi / Lh){Npo^2 (Rs + Rc)^2 + Nto^2 (Rs - Rc)^2}^0.5 = 1
or
(Pi Rc / Lh){Npo^2 ((Rs / Rc) + 1)^2 + Nto^2 ((Rs / Rc) - 1)^2}^0.5 = 1
or
(Lh / Pi Rc)
= {Npo^2 [((Rs / Rc) + 1]^2 + Nto^2 [(Rs / Rc) - 1]^2}^0.5

In a geometrically stable spheromak the quantities (Lh / Pi Rc) and (Rs / Rc) are constant, independent of the spheromak's energy.

In order to prevent Npo and Nto having a common integer factor, for Npo odd:
Npo + 2 Nto = P
, where P is a fixed prime number, characteristic of the spheromak. Hence:
dNpo = - 2 dNto

We need to find the Npo and Nto values that result in minimum spheromak energy. Hence differentiating gives:
2 Npo dNpo [((Rs / Rc) + 1]^2 + 2 Nto dNto [(Rs / Rc) - 1]^2 = 0
or
2 Npo (- 2 dNto) [((Rs / Rc) + 1]^2 + 2 Nto dNto [(Rs / Rc) - 1]^2 = 0
or
Nto [(Rs / Rc) - 1]^2 = Npo (2) [((Rs / Rc) + 1]^2

Recall that:
Npo + 2 Nto = P
or
Npo = (P - 2 Nto)
giving:
Nto [(Rs / Rc) - 1]^2 = (P - 2 Nto) (2) [((Rs / Rc) + 1]^2
or
2 P [((Rs / Rc) + 1]^2
= 4 Nto [((Rs / Rc) + 1]^2 + Nto [(Rs / Rc) - 1]^2
or
[P / Nto]
= {4 [((Rs / Rc) + 1]^2 + [(Rs / Rc) - 1]^2} / {2 [((Rs / Rc) + 1]^2}
= 2 + {[(Rs / Rc) - 1]^2 / (2 [((Rs / Rc) + 1]^2)}

Thus, if we can accurately determine (Rs / Rc) we can use this equation together with a table of prime numbers to determine P and Nto.

Note that for stable spheromaks (Rs / Rc) is a relative geometric constant independent of spheromak energy.

Recall that:
Nto [(Rs / Rc) - 1]^2 = Npo (2) [((Rs / Rc) + 1]^2
or
2 Npo / Nto = {[(Rs / Rc) - 1]^2 / [((Rs / Rc) + 1]^2}
or
4 Npo^2 = Nto^2 {[(Rs / Rc) - 1]^4 / [((Rs / Rc) + 1]^4}
giving:
(Lh / Pi Rc)
= {Npo^2 [((Rs / Rc) + 1]^2 + Nto^2 [(Rs / Rc) - 1]^2}^0.5

Note that (Lh / Pi Rc) and Nto are both spheromak geometric constants that are independent of spheromak energy.

Nto is a prime number and (Lh / Pi Rc)^2 is an integer.

Recall that:
[P / Nto]
= {4 [((Rs / Rc) + 1]^2 + [(Rs / Rc) - 1]^2} / {2 [(Rs / Rc) + 1]^2}
or
Nto
= P / ({4 [((Rs / Rc) + 1]^2 + [(Rs / Rc) - 1]^2} / {2 [(Rs / Rc) + 1]^2})
= P {2 [(Rs / Rc) + 1]^2} / {4 [(Rs / Rc) + 1]^2 + [(Rs / Rc) - 1]^2}
giving:
(Lh / Pi Rc)
= P {2 [(Rs / Rc) + 1]^2} [(Rs / Rc) - 1]
/ {2 [(Rs / Rc) + 1)] {4 [(Rs / Rc) + 1]^2 + [(Rs / Rc) - 1]^2}^0.5}}
= P {[(Rs / Rc) + 1]} [(Rs / Rc) - 1]
/ {4 [(Rs / Rc) + 1]^2 + [(Rs / Rc) - 1]^2}^0.5}

Note that stable spheromaks have a characteristic (Rs / Rc) value and hence a characteristic prime number P.

EXPLORATION OF POSSIBLE VALUES OF (Rs / Rc):
Recall that:
(Lh / Pi Rc) = P {[(Rs / Rc) + 1]} [(Rs / Rc) - 1]
/ {4 [(Rs / Rc) + 1]^2 + [(Rs / Rc) - 1]^2}^0.5}

Hence:
(Lh / Pi Rc)^2 = P^2 {[(Rs / Rc) + 1]}^2 [(Rs / Rc) - 1]^2
/ {4 [(Rs / Rc) + 1]^2 + [(Rs / Rc) - 1]^2}}

Note that since (Lh / Pi Rc)^2 is an integer then:
N = {[(Rs / Rc) + 1]}^2 [(Rs / Rc) - 1]^2
/ {4 [(Rs / Rc) + 1]^2 + [(Rs / Rc) - 1]^2}}
is an integer. Hence:
{[(Rs / Rc) + 1]}^2 [(Rs / Rc) - 1]^2
= N {4 [(Rs / Rc) + 1]^2 + [(Rs / Rc) - 1]^2}}
or
[(Rs / Rc)^2 - 1]^2 = N [4 (Rs / Rc)^2 + 8 (Rs / Rc) + 4 + (Rs / Rc)^2 - 2 (Rs / Rc) + 1]
or
[(Rs / Rc)^4 - 2 (Rs / Rc)^2 + 1] = N [5 (Rs / Rc)^2 + 6 (Rs / Rc) + 5]
or
N = [(Rs / Rc)^4 - 2 (Rs / Rc)^2 + 1] / [5 (Rs / Rc)^2 + 6 (Rs / Rc) + 5]

Try (Rs / Rc) = 2, then:
N = [9 / 37]

Try (Rs / Rc) = 3, then:
N = [81 - 18 + 1] / [45 + 18 + 5] = [64 / 68]
~ 1

Try (Rs / Rc) = 4, then:
N = [256 -32 + 1] / [80 + 24 + 5]
= 225 / 109
~ 2

Try (Rs / Rc) = 5. Then:
N = [625 -50 + 1] / [125 + 30 + 5]
= [576] / [160]
~ 4

Thus the viable choices for N are 1, 2, 3 or 4 corrresponding to (Rs / Rc) values in the range 3 to 5. These equations can be used to find exact values of (Rs / Rc ) for N = 2, 3, 4.
 

ACCURATE DETERMINATION OF (Rs / Rc):
Recall that:
[(Rs / Rc)^4 - 2 (Rs / Rc)^2 + 1] = N [5 (Rs / Rc)^2 + 6 (Rs / Rc) + 5]
or
[(Rs / Rc)^4 + (- 2 - 5 N)(Rs / Rc)^2 + [- 6 N (Rs / Rc) - 5 N + 1] = 0
or
(Rs / Rc)^2 = {(3 + 5 N) +/- [(2 + 5 N)^2 - 4 (1)(- 6 N (Rs / Rc) - 5 N + 1]^0.5} / 2
= {(3 + 5 N) +/- [(2 + 5 N)^2 + 4 (6 N (Rs / Rc) + 5 N - 1]^0.5} / 2
which can be itterated to find the exact value of (Rs / Rc)^2 and hence (Rs / Rc).

Recall that:
[P / Nto] = 2 + {[(Rs / Rc) - 1]^2 / (2 [((Rs / Rc) + 1]^2)}

Knowing the exact value of (Rs / Rc) and with the aid of a table of prime numbers we can find P and Nto.

With knowledge of both N and P we can calculate (Lh / Pi Rc)^2 using the equation:
(Lh / Pi Rc)^2 = N P^2.

Then we can find Npo using the equation:
Npo = P - 2 Nto
 

XXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXX

INNER WALL BOUNDARY CONDITION:
1) In the toroidal magnetic field region:
Bt = Muo Nt I / 2 Pi R
Note that inside the spheromak wall this field is everywhere proportional to (1 / R). Inside the sphedromak wall at R = Ro:
Bto = Muo Nt I / 2 Pi Ro

2) Inside the spheromak wall at R = Rc, Z = 0:
Btc = Muo Nt I / 2 Pi Rc.

At R = Rc, Z = 0:
Bpc = Btc
where:
Bpc = Muo Npo I / Lt
or
Npo / Lt = Nto / 2 Pi Rc
or
Npo / Nto = Lt / 2 Pi Rc

For a spheromak with a round cross section:
Lt = 2 Pi (Rs - Rc) / 2
= Pi (Rs - Rc)

Hence for a spheromak with a round cross section:
Npo / Nto = Pi (Rs - Rc) / 2 Pi Rc
= (Rs - Rc) / 2 Rc
= (1 / 2)[(Rs / Rc) - 1]

Rearranging this equation gives:
2 Rc (Npo / Nto) = (Rs - Rc)
or
Rs = Rc [1 + 2 (Npo / Nto)]
 

APPLICATION OF INNER WALL BOUNDARY CONDITION TO DETERMINATION OF (Lh / Pi Rc)

Recall that:
(Pi / Lh){Npo^2 (Rs + Rc)^2 + Nto^2 (Rs - Rc)^2}^0.5 = 1
or
(Pi / Lh){Npo^2 Rc^2 [2 + 2 (Npo / Nto)]^2 + Nto^2 (2 Rc Npo / Nto)^2}^0.5 = 1
or
(Pi / Lh){Npo^2 Rc^2 4 [1 + (Npo / Nto)]^2 + (Rc^2 Npo^2)}^0.5 = 1
or
(Pi Npo Rc / Lh){4 [1 + (Npo / Nto)]^2 + 1}^0.5 = 1
or
(Pi Npo Rc / Lh){[5 + (Npo / Nto)^2 + 2 (Npo / Nto)}^0.5 = 1
or
[5 + (Npo / Nto)^2 + 2 (Npo / Nto)} = [Lh / Pi Npo Rc]^2
or
(Npo / Nto)^2 + 2 (Npo / Nto) + [5 - (Lh / Pi Npo Rc)^2] = 0
(Npo / Nto) = {-2 + / - [4 - 4 (1)[5 - (Lh / Pi Npo Rc)^2]^0.5} / 2
or
Npo = Nto {- 1 +/- [(Lh / Pi Npo Rc)^2 - 4]^0.5}
or
Npo = Nto {- 1 + [(Lh / Pi Npo Rc)^2 - 4]^0.5}
or
(Npo + Nto)^2 = [(Lh / Pi Npo Rc)^2 - 4]
or
(Npo + Nto)^2 + 4 = (Lh / Pi Npo Rc)^2
or
Npo^2 [(Npo + Nto)^2 + 4] = (Lh / Pi Rc)^2

Since Npo and Nto are both integers then:
(Lh / Pi Rc)^2 is an integer. However, that fact is not helpful in determination of Npo and Nto because all integer values of Npo and Nto result in integer values of (Lh / Pi Rc)^2. However, knowledge of (Lh / Pi Rc)^2 imposes upper limits on Npo and Nto.

Npo^4 < (Lh / Pi Rc)^2
and
Nto^2 < (Lh / Pi Rc)^2

Recall that: Npo and Nto are prime numbers that satisfy:
2 Npo + Nto = P
or
2 Nto + Npo = P
where P = prime

Try Nto = 1, Npo = 3, both of which are prime, then:
2 Nto + Npo = 5 which is also prime

Try Nto = 2, Npo = 3, both of which are prime, then:
2 Nto + Npo = 7 which is also prime

Try Nto = 2, Npo = 7, both of which are prime, then: 2 Nto + Npo = 11, which is also prime.

Try Nto = 3, Npo = 7, both of which are prime. Then: 2 Nto + Npo = 13, which is also prime.

Thus the prime number test gives many potential Np, Nt pairs. A further test is required to select the correct Npo, Nto pair.
 

FINDING Npo AND Nto:
Recall that:
Npo^2 [(Npo + Nto)^2 + 4] = (Lh / Pi Rc)^2
where:
P = Npo + 2 Nto or
Npo^2 [(Npo + ((P - Npo) / 2))^2 + 4] = (Lh / Pi Rc)^2
or
Npo^2 [(Npo^2 + Npo (P - Npo) + (P - Npo)^2 / 4 + 4] = (Lh / Pi Rc)^2
or
Npo^2 [Npo P + [(P^2 - 2 P Npo + Npo^2) / 4] + 4] = (Lh / Pi Rc)^2
or
Npo^2 [(Npo P / 2) + ((P^2 + Npo^2) / 4) + 4] = (Lh / Pi Rc)^2
or
Npo^2 [[(Npo + P) / 2]^2 + 4] = (Lh / Pi Rc)^2

Thus it is helpful if we can quantify:
(Lh / Pi Rc)^2
which should be a large integer.
 

INNER WALL BOUNDARY CONDITION:
1) Inside the spheromak wall:
Bt = Muo Nt I / 2 Pi R
Note that inside the spheromak wall this toroidal magnetic field is everywhere proportional to (1 / R). Inside the spheromak wall at radius R:
Bt = Muo Nt I / 2 Pi R

2) Inside the spheromak wall at R = Rc, Z = 0:
Btc = Muo Nt I / 2 Pi Rc.

3) Inside the spheromak wall at R = Rs:
Bts = Muo Nt I / 2 Pi Rs
 

INNER WALL BOUNDARY CONDITION:
Due to spheromak symmetry, for R < Rc on the equatorial plane where Z = 0 the electric field R and Z components all cancel. Hence at R= Rc, Z = 0 the poloidal magnetic field energy density at the inner spheromak wall equals the toroidal magnetic field energy density at the inner spheromak wall. Thus:
[Bp|(R = Rc)]^2 / 2 Muo
= [Bt|(R = Rc)]^2 / 2 Muo
or
[Bp|(R = Rc)] = [Bt|(R = Rc)]
or
(Muo Np I / Lt) = Muo Nt I / 2 Pi Rc
or
Np / Lt = Nt / 2 Pi Rc
or
Np / Nt = Lt / 2 Pi Rc

This result is the inside wall boundary condition. Note that this inner wall boundary condition is independent of charge Q.
 

OUTER WALL BOUNDARY CONDITION:
Similarly, at the outer wall of the spheromak on the equatorial plane the sum of the external poloidal magnetic field energy density and the external radial electric field energy density equals the internal toroidal magnetic field energy density inside the spheromak wall. Thus:
[Bp|(R = Rs)]^2 (1 / 2 Muo) + (Epsilono / 2) [Er|(R = Rs)]^2
= (1 / 2 Muo) [Muo Nt I / 2 Pi Rs]^2

Note that Er contains electric field contributions from both the inner and outer spheromak walls.

The spheromak's poloidal magnetic field energy density decays rapidly with increasing distance from the spheromak center in proportion to (1 / R^6) but the spheromak's electric field energy density extends to infinity decaying in proportion to (1 / R^4) for R >> Ro.
 

This web page last updated September 26, 2020.