XYLENE POWER LTD.

SPHEROMAKS - INTRODUCTION

By Charles Rhodes, P.Eng., Ph.D.

SPHEROMAK OVERVIEW:
This web page introduces basic spheromak concepts.

Nature stores localized energy in electromagnetic structures known as spheromaks. In general, each quantum charged particle is a spheromak. Spheromks have ground states and various excited states. The extent of average spheromak excitation is determind by its radiation environment. At equilibrium the rate of radiation emission by a sheromak is equal to its rate of radiation absorpion.

The properties of a spheromak may be investigated by exciting the spheromak and then observing its radiative decay.

A spheromak is a naturally occurring electromagnetic structure that enables stable amounts of charge and field energy such as quantum charged particles and atoms. There are also semi-stable plasma spheromaks. A spheromak is a stationary solution to Maxwells equations of electromagnetism. A spheromak consists of a filament of circulating net charge that spontaneously forms a quasi-toroidal shaped closed wall that divides the local space into two regions. Inside this closed wall the vector field is purely toroidal magnetic. Outside this closed wall the vector field is mixed poloidal magnetic and radial electric. The vector fields contain energy that provides rest mass.

A spheromak has cylindrical symmetry around its major Z axis and is mirror symmetric about its equatorial plane at Z = 0.

At the spheromak wall, on both sides of a spheromak wall the total field energy densities are equal.

The average field energy density inside the spheromak wall is less than the average field energy density outside the spheromak wall, which causes a spheromak to be a potential energy well. This potential energy well gives a spheromak its stability.

The vector fields each contribute to spheromak energy and hence spheromak rest mass. If the circulating charge consists of multiple separate quantum charged particles, they also each contribute rest mass to the assembly.

Spheromaks are used by nature to form stable local concentrations of charge and electromagnetic field energy. Spheromaks are instrumental in nuclear and atomic particle interactions. Spheromaks also have important roles in semi-stable plasmas, chemical binding and thermal radiation.
 

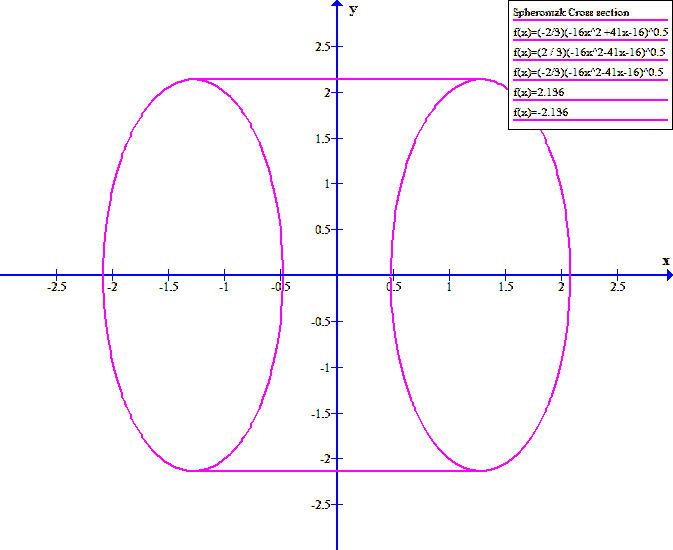
SPHEROMAK WALL DIAGRAM:
The following diagram shows the approximate cross sectional shape of a simple theoretical spheromak wall in free space.

The spheromak wall outside radius on its equatorial plane is:
R = Rs,
the spheromak wall inside radius on its equatorial plane is:
R = Rc,
and the maximum height of the spheromak wall is:
Z = Hm.
 

SPHEROMAK CONCEPT:
Conceptually a spheromak is a wound charged filament in the shape of a quasi-toroid which provides a closed path for the current in the charged filament. The direction of the charged filament axis conforms to the spheromak wall surface curvature.

The current path through the charged filament that forms a spheromak has both toroidal and poloidal magnetic field components. The spiral charge filament axis changes direction over the surface of the quasi-toroid. The toroidal magnetic field has two possible directions with respect to the poloidal magnetic field.

In a spheromak filament positive and negative charge quanta move along a spiral path that is continuously tangent to the spheromak wall. A spheromak is cylindrically symmetric about the spheromak main axis and is mirror symmetric about the spheromak's equatorial plane. The spheromak wall has a net charge Qs that is uniformly distributed over the filament length Lh. Hence the surface charge density near the inner perimeter of the spheromak wall is larger than near the outer perimeter of the spheromak wall.

In the center of the spheromak at R = 0 and Z = 0 the electric field cancels to zero. In the region inside the spheromak wall the magnetic field is purely toroidal and the electric field is zero. Outside the spheromak wall the magnetic field is purely poloidal and the electric field is radial. The net charge circulates at the speed of light along the current path of length Lh within the thin spheromak wall at the boundary between the toroidal and poloidal magnetic field regions.
 

STABLE SPHEROMAK:
In a spheromak the motion of the positive and/or negative quantum charges along the charge filament causes filament current I and hence poloidal and toroidal magnetic fields. The net charge on the charge filament produces the external electric field outside the spheromak wall and cancels the electric field inside the spheromak wall. The magnetic force between adjacent charge filament turns balances the electric force between adjacent charge filament turns, allowing the charge filament to form a stable closed path that is the spheromak wall. The spheromak wall shape and circulating current configuration are such that inside the spheromak wall the net poloidal magnetic field is zero.
 

SUMMARY:
A charged filament forming a spheromak is characterized by a net charge Qs, a net filament current I, a stored static electromagnetic energy Ett, and a filament length Lh. Isolated spheromaks in a vacuum have a characteristic geometry and characteristic frequency:
Fh = C / Lh.
The circulating charge motion gives the spheromak an external poloidal magnetic field and an internal toroidal magnetic field. Note that the internal toroidal magnetic field has two possible directions with respect to the external poloidal magnetic field.
 

SPHEROMAK APPLICATIONS:
Spheromaks occur as a result of electric charge circulation within quantum charged particles, as a result of electron circulation in atoms, molecules and crystals, as a result of free electron circulation in metals and as a result of electron and ion circulation in plasmas. Spheromaks are also involved in the charged particle photon absorption and emission that occurs on application of an external magnetic field and ass a result of thermal excitation of gases.

An isolated spheromak has a unique energy state solution. An isolated spheromak subject to an external magnetic field has two discrete energy states. When there is a suitable applied external magnetic field spheromaks can jump between the two discrete energy states by absorption or emission of an electromagnetic photon of the appropriate frequency.

This phenomena is variously known as nuclear magnetic resonance (NMR) and electron spin resonance. NMR of hydrogen atoms in body tissue is widely used for medical imaging.

In situations where there are multiple interacting spheromaks there is a spectrum of discrete energy state solutions.

Hot gas atoms also absorb or emit electromagnetic photons at various characteristic frequencies. That is the basis of gas spectroscopy.

In an experimental apparatus known as a Kibble Balance the Planck constant h is reproducible to many significant figures, so today this experimentally measured constant is used to relate international standard units of energy (mass) to international standard units of time (frequency).
 

FURTHER SPHEROMAK ATTRIBUTES:
A free neutron is thought to be a dual spheromak assembly with no net charge. In a nuclear reactor after a few minutes a free neutron will spontaneously decay into a proton, an electron and a neutrino. However, in an atomic nucleus, which imposes external electric and magnetic fields, a neutron is often very stable.

Spheromaks can electrically and magnetically bind together and/or merge to form atomic assemblies with larger rest energy.

The quantum electron charges around an atomic nucleus form multi-particle spheromaks. About half of these quantum electron charges move poloidally opposite to the other half to minimize the net poloidal magnetic field.

The spheromak structure of atomic electrons explains experimentally observed atomic ionization energies and chemical bonding.

Nucleons tend to arrange themselves so that their constituant poloidal magnetic fields cancel.

The spheromak mathematical model allows precise calculation of the contribution of particle electric and magnetic field energies to electron and proton rest masses.

Plasma spheromaks are used for energy and fuel injection in some nuclear fusion processes. "Ball Lightning" is an occasionally observed form of plasma spheromak.
 

The spheromak mathematical model predicts the experimentally measured Planck constant h, and the corresponding Fine Structure constant Alpha which are fundamental to quantum mechanics. Generally in any physical process that exhibits energy quantization in accordance with the Planck constant there is an underlying spheromak.

SPHEROMAK EXISTENCE:
Plasma spheromaks have been photographed, so there is no doubt as to their existence.

A spheromak is a stable distorted toroid shaped electromagnetic structure that naturally forms as a result of a net charge Qs continuously circulating at the speed of light C forming current I that flows in a multi-turn closed filament of length Lh. The net charge Qs is uniformly distributed along the filament length Lh.

Spheromaks form local potential energy wells.

Basic electromagnetic theory indicates that electric currents flowing in the same direction in parallel filaments magnetically attract each other. If these parallel filaments have the appropriate net charge per unit length adjacent current filaments electrically repel each other. In circumstances where the electric and magnetic forces on the adjacent current filaments are in precise balance a spheromak can exist. This spheromak existence requirement is developed on the web page titled CHARGE FILAMENT PROPERTIES.
 

MATHEMATICAL MODEL OF A SPHEROMAK:
On this web site spheromak field energy density functions are developed in terms of spheromak geometrical size, number of poloidal filament turns Np, number of toroidal filament turns Nt, charge and current parameters. The spheromak field energy density functions are shown to yield spheromaks with known static electric and magnetic field energy content. Hence the total spheromak static electric and magnetic field energy is expressed in terms of measureable parameters. It is shown that quantum mechanical properties, such as the Planck constant and Fine Structure constant, in combination with quantization of charge, arise from these parameters.

The focus of the spheromak mathematical model developed on this web site is on practical engineering issues such as relationships between spheromak linear size, spheromak geometrical shape, spheromak net charge, natural frequency, spheromak poloidal and toroidal magnetic field strengths, spheromak electric field strength, spheromak total field energy, plasma spheromak circulating electron kinetic energy, the number of free electrons in a plasma spheromak, the plasma spheromak enclosure size and plasma spheromak lifetime. The result is a practical mathematical model that gives relatively simple closed form solutions to problems that might otherwise likely require extensive computing power.

The utility of the spheromak mathematical model is demonstrated by comparison of predictions from the spheromak mathematical model to experimental data. Spheromaks account for many experimentally observed quantum mechanical phenomena.
 

APPROACH:
In most introductory physics courses electricity and magnetism are taught from a point force perspective. However, dealing with spheromaks from a point force perspective is mathematically difficult. It is mathematically much simpler to recognize that a force is the result of a change in field potential energy density with respect to position and deal with spheromaks from a potential energy density perspective.

It is likely helpful for the reader to grasp the electromagnetic principles set out on the web page titled CHARGE FILAMENT PROPERTIES before moving on to study the structure and energy content of a spheromak.
 

SPHEROMAK GEOMETRY:
A spheromak has a stable distorted toroid shaped filament winding carrying current I with net charge Qs continuously circulating around a closed path of length Lh at the speed of light C. The filament's net charge Qs is uniformly distributed along the filament length Lh.

The toroid surface, herein referred to as the spheromak wall, contains a blend of Np poloidal turns around the spheromak major axis of symmetry and Nt toroidal turns around the spheromak minor axis.

The spheromak wall separates the region inside the spheromak wall from the region outside the spheromak wall.

The circulating current forms a purely toroidal magnetic field inside the spheromak wall and a poloidal magnetic field outside the spheromak wall.

The net electric charge Qs on the filament causes a radial electric field outside the spheromak wall. Inside the spheromak wall the electric fields cancel so that inside the spheromak wall the net electric field is zero.

At the exact center of the spheromak, due to spheromak symmetry, the electric fields also cancel to zero and the magnetic field is purely poloidal and axial.

Outside the spheromak wall there are both a poloidal magnetic field and a radial electric field but the toroidal magnetic field is zero.

Hence a stable isolated spheromak has external radial electric field, external poloidal magnetic field and internal toroidal magnetic field energy components.

The toroidal magnetic field in a spheromak may either clockwise (CW) or counter clockwise (CCW) with respect to the spheromak's poloidal magnetic field.

An isolated spheromak retains its size, shape and energy due to its own electric and magnetic fields. The spheromak wall is located on the locus of points where the total field energy densities on both sides of the spheromak wall are exactly equal. The spheromak wall position is stable because the second derivatives of total spheromak field energy with respect to spheromak wall position are positive everywhere at the spheromak wall.

A spheromak has a characteristic radius Ro. On loss of spheromak energy the characteristic radius of an isolated spheromak increases but the relative spheromak geometry remains unchanged.

A spheromak forms a potential energy well because the average toroidal magnetic field energy density within the spheromak wall is less than the sum of the average radial electric and poloidal magnetic field energy densities outside the spheromak wall.

The net charge and the charge motion along the closed filament current path cause the forces on each circulating quantum charge to net to zero. Note that inertial forces and relativistic phenomena apply to plasma spheromaks but do not affect quantum charged atomic particle spheromaks.

Spheromak parameters, geometry and the spheromak potential energy well are further discussed on other web pages attached hereto.
 

SPHEROMAK WALL:
The spheromak wall separates the toroidal magnetic field region enclosed by the spheromak wall from the poloidal magnetic and radial electric field region outside the spheromak wall. Hence a stable isolated spheromak has radial electric, poloidal magnetic and toroidal magnetic field energy components.

As shown at Charge and Current Filament Behaviour for a stable spheromak wall the filament current I is given by: I = Qs C /Lh = Rhoh C

At every point on the spheromak wall there is perfect balance between the local internal toroidal magnetic field energy density and the sum of the local external field energy densities. The position of this field energy density balance sets the position of the spheromak wall.

Due to a combination of electromagnetic field theory, spheromak wall geometry and prime number theory only certain values of number of toroidal filament turns Nto and number of poloidal filament turn Npo can exist in a stable spheromak.

Since the inner spheromak wall and the outer spheromak wall have different radii with respect to the spheromak main axis of symmetry, at the inner wall the separation between adjacent current filaments is less than at the outer wall. Hence the surface electric field is larger at the inner wall than at the outer wall.
 

SPHEROMAK GEOMETRY:

Let R be the radial distance of a point from the main axis of symmetry of a spheromak.

Let Z be the height of a point above the equatorial plane of a spheromak.

SPHEROMAK STRUCTURE:
The geometry of a spheromak is symmetric about its central point:
R = 0, Z = 0
and can be characterized by:
its outer radius R = Rs at Z = 0 ,
its inner radius R = Rc at Z = 0
and its height Z = +/- Ho at R = Ro

For the stable spheromak geometry the ratios (Ho / Ro), (Rc / Ro) and (Rs / Ro) are constants irrespective of the value of Ro.

Note that a theoretical round spheromak with a minor axis at:
Ro = (Rs + Rc) / 2, Z = 0
has a spheromak wall position defined by:
{Z^2 / Ho^2} + {[R - ((Rs + Rc) / 2)]^2 / [(Rs - Rc) / 2]^2} = 1.

It is shown that the total electromagnetic field energy Ett trapped by a spheromak is proportional to (1 / Lh) where:
Lh^2 = (Np Lp)^2 + (Nt Lt)^2
Lp = 2 Pi Ro
Lt = 2 Pi K Ro = length of one winding turn around the toroidal magnetic path

Current moves along the spheromak closed path length Lh at speed of light C. Hence the natural frequency F of the path length Lh is:
F = C / Lh.

A spheromak's total field energy is Ett.
Ett / F = Ett Lh / C = h

Expressions for Ett typically contain the factor (1 / Lh) so that calculations of (Ett Lh / C) result in the constant h.

Hence:

Hence, Ett is proportional to frequency F.
Ett = h F
The proportionality constant is known as the Planck constant h

Thus spheromaks are the cause of photon energy quantization.
 

CONSTANT CHARGE FILAMENT CURRENT:
An important issue in spheromak analysis is that the charge filament current I is the same everywhere on the charge filament.

CHARGE FILAMENT GEOMETRY:
Define:
Lt = one purely toroidal filament turn length;
Lp = one average purely poloidal filament turn length;
Np = number of poloidal turns
Nt = number of toroidal turns
When an element of charge has passed through the spheromak core Nt times it has also circled around the main axis of spheromak symmetry Np times, by which time it reaches the point in the closed filament path where it originally started.

There are Nt parallel filament turns that go through the equatorial plane in the central core of the spheromak.
 

DISCRETE INTEGER SOLUTIONS:
There is a further aspect of charge filaments that is important. In order for a spheromak to be stable over time each circuit of the charge filament must be identical to every other such circuit. Hence for the spheromak to be stable the number of toroidal turns Nt and the number of poloidal turns Np included in length Lh must both be integers.
 

SPHEROMAK NET CHARGE:
Net charge Qs on the spheromak is given by:
Qs = Qp Nph + Qn Nnh
 

SPHEROMAK FIELD ENERGY DENSITY FUNCTIONS:
A spheromak has three cylindrically symmetric field energy density functions:
Ut(R,Z) = toroidal magnetic field energy density function
Up(R,Z) = poloidal magnetic field enegry density function
Ue(R,Z) = radial electric field energy density function

The spheromak has two regions which are separated by the thin closed spheromak wall.

Everywhere in the region inside the spheromak wall the field energy density U is given by:
U = Ut(R, Z)
where:
Ut(R, Z) < Up(R, Z) + Ue(R,Z)

Everywhere in the region outside the spheromak wall:
U = Up(R,Z) + Ue(R, Z)
where:
Ut(R, Z) > Up(R, Z) + Ue(R, Z)

Hence the spheromak forms a potential energy well.

Everywhere on the spheromak wall:
Ut(R, Z) = Up(R, Z) + Ue(R,Z).
Ths equation defines the position of the spheromak wall.
 

SPHEROMAK SYMMETRY:
Spheromaks exhibit mirror symmetry about the equatorial plane. Hence:
Z(R) = - Z(R)
and U(R, Z) = U(R, - Z)

Hence:
Ett = Integral from Z = - infinity to Z = + infinity of:
Integral from R = 0 to R = infinity of:
U(R, Z) 2 Pi R dR dZ
= Integral from Z = 0 to Z = infinity of:
U(R, Z) 4 Pi R dR dZ
 

SPHEROMAK WALL POSITION:
The position of the spheromak wall corresponds to the lowest available spheromak energy state. At this state the spheromak energy density distribution is U(R, Z) where:
inside the spheromak wall:
U = Ut(R)
and outside the spheromak wall:
U = Up(R, Z) + Ue(R,Z)
and at the spheromak wall:
Ut(R) = Up(R, Z) + Ue(R,Z)
 

ISOLATED SPHEROMAKS:

Spheromaks are used by nature to form particles that are local concentrations of charge and electromagnetic field energy. We commonly refer to this locally concentrated electromagnetic field energy as rest mass. Spheromaks are instrumental in nuclear and atomic particle interactions. Spheromaks also have important roles in semi-stable plasmas, chemical binding and thermal radiation.

An isolated spheromak has a unique energy state solution. An isolated spheromak in an external magnetic field has two energy state solutions. When there are multiple interacting spheromaks there is a spectrum of discrete energy state solutions.

Spheromaks gain or lose energy by absorption or emission of electromagnetic radiation.
 

BOUNDARY CONDITIONS:
For an isolated charged particle spheromak in a vacuum the electric field inside the spheromak wall is zero.

For an isolated spheromak the field energy density outside the wall is the sum of the energy densities caused by the electric field due to fixed charge Qs and by the polodal magnetic field arising from current circulation. The field energy density inside the spheromak wall is caused only by the toroidal magnetic field that arises from toroidal current circulation in the spheromak wall.

Thus, from basic electromagnetic theory inside the spheromak wall the toroidal magnetic field is given by:
Bt = Muo Nt I / 2 Pi R so inside the spheromak wall the toroidal magnetic field energy density is given by:
Ut = Bt^2 / 2 Muo
= [Muo Nt I / 2 Pi R]^2 / 2 Muo
= Muo Nt^2 I^2 / 8 Pi^2 R^2
where:
Muo = permiability of free space = 4 Pi X 10^-7____
Pi = 3.14159265
Nt = number of toroidal turns
I = circulating current
R = the radial distance from the main axis of spheromak symmetry, herein referred to as the Z axis.

This region inside the spheromak wall exists within a larger region outside the spheromak wall where the magnetic and electric field energy densities are approximately equal to the magnetic and electric fields produced by a current ring located at R = Ro, Z = 0 carrying a current (Np I) and having net charge Qs.
 

SPHEROMAK STABILITY:
Spheromak stability arises from prime number constraints that affect Np and Nt as well as from formation of a potential energy well inside the spheromak wall. Due to a combination of electromagnetic field theory, spheromak geometry and prime number theory only certain values of Nt and Np can exist in a stable spheromak.

The integers Np and Nt cannot be equal and cannot have a common factor other than unity. As a result of Np and Nt having no common factors the filament current path (winding) has only one layer with no cross overs. The filament only intersects itself at the current path closure point. Spheromak stability suggests that small errors in Np or Nt should lead to self correction rather than spheromak collapse.

Apart from the constraints on Np and Nt detailed analysis shows that spheromaks are stable due to formation of an energy well inside the spheromak wall.

The optimum spheromak geometry is stable over a wide range of spheromak energy. The spheromak wall relative gepmetry remains constant and can be geometrically characterized by the size parameters Ro. For a stable spheromak the ratios: (Ho / Ro), (Rc / Ro) and (Rs / Ro) remain constant independent of the spheromak's total field energy.

The stable spheromak structure enables the existence of isolated charged particles and semi-stable plasmas. Particles with rest mass are stable packets of charge and energy that are non-propagating solutions to electromagnetic equations. Hence atoms and charged atomic particles embody spheromaks.
 

SPHEROMAK WINDING:
The spheromak winding has Npo poloidal turns and Nto toroidal turns. The numbers Np and Nt are positive integers with various special mathematical properties.

The winding length Lh is given by:
Lh^2 = (Np Lp)^2 + (Nt Lt)^2
= [Np 2 Pi Ro]^2 + [Nt 2 Pi (Rs - Rc) / 2]^2
where:
Lp = 2 Pi Ro
is the length of a single poloidal filament winding turn and Lt = 2 Pi (Rs - Rc) / 2
? is the length of a filament winding turn that encircles the toroidal magnetic flux once.

The spheromak inner wall and the spheromak outer wall have different radii with respect to the main axis of symmetry. Hence, at the inner wall the current filament to current filament distance is less than at the outer wall. Since the net charge is evenly distributed along the filament this issue causes the surface charge per unit area at the outer wall to be less than the surface charge per unit area at the inner wall.

The current filament turns occur in a single layer which contains no cross overs. The current path only intersects itself at the current path closure point. The spheromak must remain stable in the presence of small geometric disturbances that can cause either Np or Nt to increment or decrement from their nominal Np = Npo and Nt = Nto values. Hence, in a stable spheromak, Npo and Nto do not share common factors. Similarly there is no common factor sharing between: Npo + 2, Nto - 1; between Npo - 2 and Nto + 1, between Npo + 4 and Nto -2 and between Npo - 4 and Nto + 2.

In a spheromak Npo cannot equal Nto.

Spheromak stability is enhanced if a small energy disturbance does not lead to spheromak collapse. Ideally, in addition to P being prime, Npo and Nto are both prime numbers. Then if there is a disturbance that affects only Np or only Nt the spheromak remains stable.
 

Any point on the spheromak winding can be identified by its Phi and Theta values. Phi is the angle about the major axis of symmetry measured with respect to a radial line from the minor axis to the orgin. Theta is the angle about the minor axis at R = Ro, Z = 0 measured with respect to the same radial line. Hence at the current path closure point:
Phi = 0, Theta = 0 or Phi = 2 Pi Np, Theta = 2 Pi Nt

The range of Phi is:
0 < Phi < 2 Pi Npo radians;
The range of Theta is:
0 < Theta < 2 Pi Nto radians

The average value of dTheta / dPhi is given by:
dTheta / dPhi = 2 Pi Nto / 2 Pi Npo
= Nto / Npo.
However, in a spheromak dTheta / dPhi varies along the winding and is a function of R.

Symmetry indicates that at the center of the spheromak core the net electric field is zero and the net toroidal magnetic field is zero.
 

SPHEROMAK TOTAL FIELD ENERGY:
The spheromak total field energy given by:
Ett = Integral over all space of:
U(R, Z) 2 Pi R dR dZ

Spheromaks are used by nature to form particles that are local concentrations of electromagnetic field energy. We commonly refer to this locally concentrated electromagnetic field energy as rest mass. Spheromaks are instrumental in nuclear and atomic particle interactions. Spheromaks also have important roles in semi-stable plasmas, chemical binding and thermal radiation.

An isolated spheromak has a unique energy state solution. An isolated spheromak in an external magnetic field has two energy state solutions. When there are multiple interacting spheromaks there is a spectrum of discrete energy state solutions.

Spheromaks gain or lose energy by absorption or emission of electromagnetic radiation.
 

NATURAL FREQUENCY:
The spheromak wall consists of a long filament of charge that forms a complex closed spiral current path with length Lh. This current path contains Nt toroidal turns and Np poloidal turns. The numbers Np and Nt have no common factors so the current path never crosses itself. The current flows along this path at the speed of light. The length of this current path and the speed of light together give the spheromak a characteristic frequency Fh.

The natural frequency Fh given by:
Fh = C / Lh.

It is shown on this web site that:
dEtt / dFh = h,
where:
h = Planck Constant

In certain circumstances a stable atomic particle spheromak can absorb quantum amounts of electromagnetic radiation (photons) with energy Ep = h Fp where:
Ep = photon energy
h = Planck constant
Fp = photon frequency

The change in the total field energy content of a spheromak dEtt is proportional to its change in frequency dFh.

The PLANCK CONSTANT h is a combination of physical constants that arise from the geometry of a quantum charged spheromak.
 

CHARACTERISTIC FREQUENCY:
In an atomic particle the time required for movement of net charge:
(Qp Nph + Qn Nnh)
at velocity C around the closed charge filament path of length Lh gives the spheromak a characteristic frequency Fh where:
Fh = C / Lh

For a given net electric charge Qs the smaller a spheromak is the more total energy Ett that it traps and the higher is its characteristic frequency Fh. If an atomic particle spheromak's energy changes due to photon capture or photon emission while the spheromak net charge Qs remains constant there is a corresponding change in spheromak size and hence there is a corresponding change in the spheromak characteristic frequency Fh. An emitted or absorbed photon must reflect both the change in total spheromak energy
(Ettb - Etta)
and the change in the spheromak characteristic frequency
(Fhb - Fha).
Note that the emitted or absorbed photon frequency is at the beat frequency difference between the initial spheromak frequency Fha and the final spheromak frequency Fhb.
Expressed mathematically:
(Ettb - Etta) = h (Fhb - Fha)
= h [(C / Lhb) - (C / Lha)]

Provided that the spheromak relative geometry remains constant:
Lhb / Lha = Rob / Roa
 

PLANCK CONSTANT:
A quantum charged particle is itself a highly stable spheromak.

Spheromaks gain and lose radiant energy in accordance with:
dE = h F
where:
dE = the photon energy absorbed or emitted by the spheromak;
h = Planck constant;
F = C / Lh
where:
F = natural frequency of the spheromak;
C =speed of light;
and
Lh = the circulating charge filament path length.

Thus the Planck constant is really a manifestation of spheromak electromagnetic field geometry and quantum charged spheromaks provide the underlying mechanism of photon energy quantization.

Spheromaks are fundamental to atomic particle physics. Spheromaks also have important roles in semi-stable plasmas, chemical binding, thermal radiation and gas spectroscopy.
 

RADIATION AND MATTER:
A spheromak is a mathematical representation of an isolated charged particle. Electro-magnetic spheromaks are stable energy states. These stable states are reached by emission or absorption of radiation. During radiant energy emission and absorption total system energy and total system momentum are conserved. Charged particles and radiation, both have characteristic frequencies. During photon emission the emitting spheromak's frequency Fh decreases and the amount of propagating radiant energy increases. During photon absorption the absorbing spheromak's natural frequency Fh increases and the amount of propagating radiant energy decreases.

A spheromak has an electric current which follows a closed spiral path that traces out the shape of the wall of a quasi-toroid. The closed current path has both has both toroidal and poloidal circulation components. The quasi-toroidal surface is referred to as the spheromak wall.

The current circulates at the speed of light. At the spheromak geometry the total field energy density just inside the spheromak wall equals the total field energy density just outside the spheromak wall. Hence the electric and magnetic forces are in balance everywhere on the spheromak wall making the spheromak geometrically stable.

The existence, mass and other properties of each spheromak and hence each real atomic particle is governed in part by the prime number P that simultaneously satisfies all of the spheromak constraint equations. Since P, and the spheromak winding parameters Np and Nt must be integers and Np and Nt cannot share common factors the number such prime numbers and hence the number of real atomic particle possibilities is distinctly limited.

If a spheromak's static electromagnetic field energy Ett changes from Ea to Eb and the spheromak frequency Fh changes from Fa to Fb then:
dEtt = (Ea - Eb)
= h (Fa - Fb)
= h dFh

Over time spheromaks in free space will absorb or emit energy until they reach a stable state.
At this stable state the value of (dEtt / dFh) for an electromagnetic spheromak is given by:
(dEtt / dFh) = h, where:
Fh = the natural frequency of the circulating quantum net charge that forms an electromagnetic spheromak and dFh is the frequency of a radiation emitted or absorbed.

This formula is the basis of quantum mechanics. Spheromaks form the static field structure of charged particles with rest mass. Since spheromaks are the main sources and sinks of radiant energy, spheromak properties in large measure determine the radiant energy absorption and emission properties of matter.

SPHEROMAK TRANSIENT BEHAVIOR:
When a spheromak first forms the spheromak will emit photons in order to reach its most stable energy state at which state it is in radiation balance with its environment. In a low radiation environment that is the spheromak minimum energy or ground state.

The electrons surrounding an atomic nucleus form spheromaks with nearly cancelling poloidal magnetic fields.
 

NO RADIATION IN THE GROUND STATE:
An important property of an isolated charged particle spheromak is that in its minimum energy state, also known as its ground state, the spheromak does not emit radiation. This property enables the existence of stable quantum charged particles, stable atomic nuclei and stable atoms. Note that as a spheromak approaches it ground state its radiation emission becomes very small. Possibly much of the cosmic background radiation is due to gradual spheromak energy decay. That low level radiation will only cease when the prevailing temperature is at absolute zero.
 

RELATED MATERIAL ON THIS WEB SITE:
At other web pages on this web site spheromak energy density functions are developed in terms of spheromak geometrical size, poloidal and toroidal turns, charge and current parameters. The spheromak energy density functions are shown to yield spheromaks with known static electric and magnetic field energy content. Hence the total spheromak static electric and magnetic field energy is expressed in terms of measureable parameters. It is shown that quantum mechanical properties, such as the Planck constant and Fine Structure constant, arise from these parameters.

The utility of the speromak mathematical model is demonstrated by comparison of predictions from the spheromak mathematical model to experimental data. Spheromaks account for most experimentally observed quantum mechanical phenomena.

The result is a practical mathematical model that gives relatively simple closed form solutions to problems that would otherwise likely require extensive computing power.

Other spheromak issues include: plasma spheromak circulating electron kinetic energy, the number of free electrons in a plasma spheromak, the plasma spheromak enclosure size and plasma spheromak lifetime.
 

This web page last updated August 27, 2024.